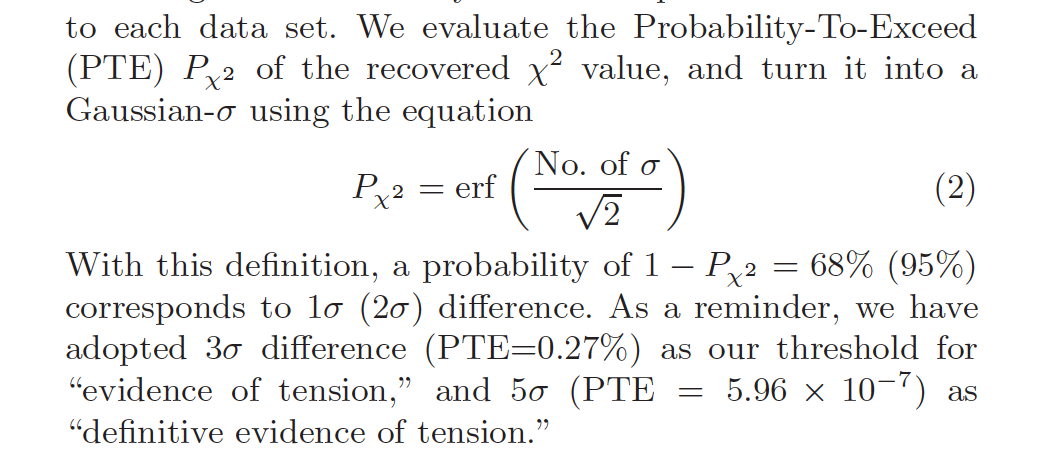
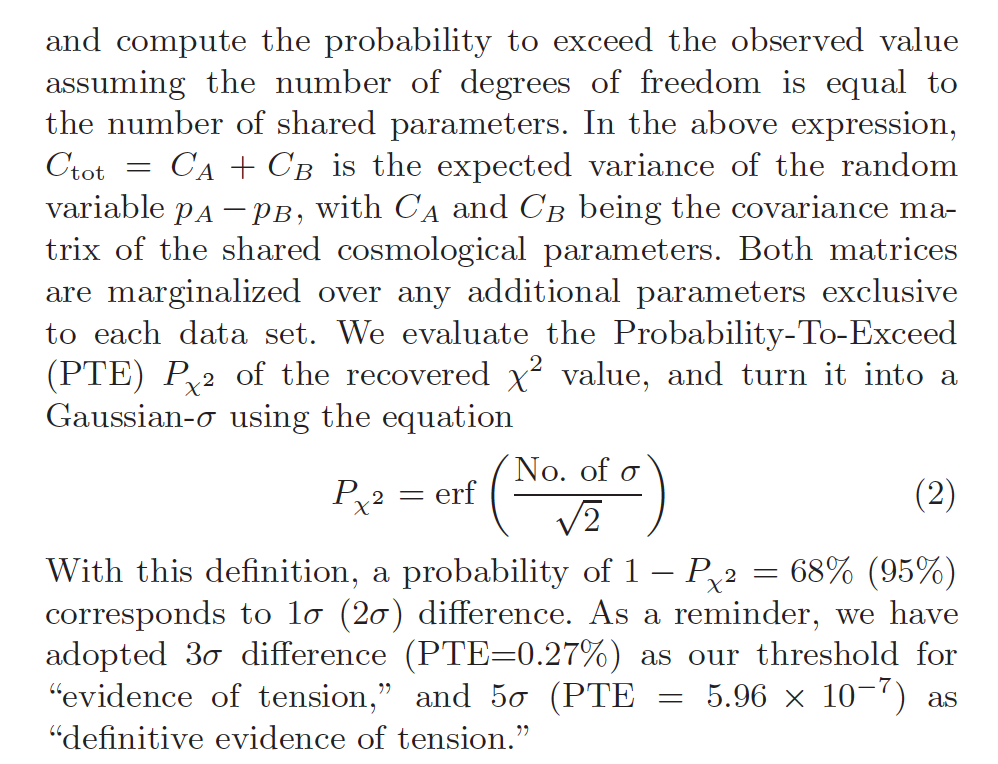
I would like to understand the difference between the $\chi^{2}$ distribution and the Probability-To-Exceed ?
I have to compare 2 data sets A and B and in the article I am reading, they talk about this PTE :
I only know the $\chi^{2}$ distribution with $k=2$ degrees of freedom :
$$f(\Delta\chi^{2})=\dfrac{1}{2}\,e^{-\dfrac{\Delta\chi^{2}}{2}}\quad(1)$$
and the relation with confidence level :
$$1-CL={\large\int}_{\Delta\chi^{2}_{CL}}^{+\infty}\,\dfrac{1}{2}\,e^{-\dfrac{\Delta\chi^{2}}{2}}\,d\,\chi^{2}=e^{-\dfrac{\Delta\chi_{CL}^{2}}{2}}\quad(2)$$
I don't know how to do the link with the text above.
In the article, they make appear the integral of gaussian whereas in $(2)$, I can only make appear a simple integration of exponential (I mean, there is no "$\text{erf}$" function appearing unlike into the article).
If someone could tell me the difference between $\chi^{2}$ distribution and $P_{\chi^2}$ (PTE) ?
UPDATE 1: the context is about astrophysics where I have to compare the consistency of 2 data sets (cosmological parameters) . The method is described below :
Could anyone tell me what's the definition of this Probability-To-Exceed and how to determine it ?
Is it a cumulative function ? How to get the integral of a gaussian in this case (since erf appears) ?
Any help is welcome, regards