On page 86 of Causal Inference in Statistics: A Primer, by Pearl, Glymour, and Jewell, the authors state that
A variable is called an "instrument" if it is $d$-separated from $Y$ in $G_\alpha$ and, it is $d$-connected to $X.$
Note here that $G_\alpha$ is the graph obtained from the initial causal graph by removing the arrow from $X$ to $Y.$ These conditions are different from your #1 assumption, as correlation might be there or it might not. The quoted condition is equivalent to your #2. Without this condition, the variable would not be an instrument, and the ensuing analysis would be invalid.
In the case of the death of the highly productive worker, surely that is a mediation situation, like this:
[![enter image description here][1]][1]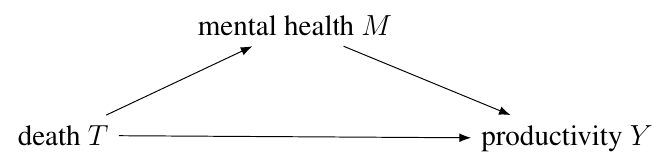
Nothing in sight could be used as an instrumental variable, though you definitely could use mediation analysis. [1]: https://i.sstatic.net/UL0fv.png
