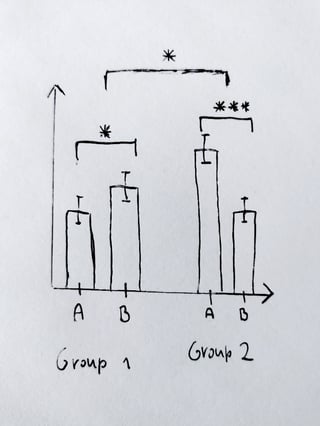
Let's say there are two groups of participants. Each group is randomly split into control subgroup A and task subgroup B. For each of the 4 cases some random variable $x$ is measured, whose mean is denoted by $\mu$. I would like to perform 3 tests:
- $H_0 : \mu_{A, Group1} \neq \mu_{B, Group1}$$H_0 : \mu_{A, Group1} = \mu_{B, Group1}$
- $H_0 : \mu_{A, Group2} \neq \mu_{B, Group2}$$H_0 : \mu_{A, Group2} = \mu_{B, Group2}$
- $H_0 : \mu_{Group1} \neq \mu_{Group2}$$H_0 : \mu_{Group1} = \mu_{Group2}$ regardless of subgroups
I sketch the tests below
Question: What is the correct way to perform these tests? If I perform 3 independent t-tests, is it fair to apply Bonferroni correction to their p-values, or must I do a more sophisticated multiple comparisons correction. If making independent tests is not a robust procedure, what is? I want to do all 3 tests, so as far as I understand I don't want two-way ANOVA.