I have data where each person can take 5 potential values of $(0, 0.25, 0.5, 0.75, 1)$. Each person is part of a larger group of 3 people, so that the reported value for each group is the average of 3 people's values, which is between 0 and 1.
For example,
Group Name Scores Average of Scores
Group 1 (0.50, 0, 0.50) 0.333
Group 2 (1.0, 0.5, 1.0) 0.833
Group 3 (0.75, 0.5, 0.25) 0.500
Group 4 (0.5, 1, 0.5) 0.666
I would like to construct a confidence interval for the "Average of Scores" column. Is it fair to use a confidence interval for binomial proportion $\hat{p}=(0.333+0.833+0.5+0.666)/4$
$$ \hat{p} \pm z_{\alpha}\sqrt{\dfrac{p(1-p)}{N}} $$ ?
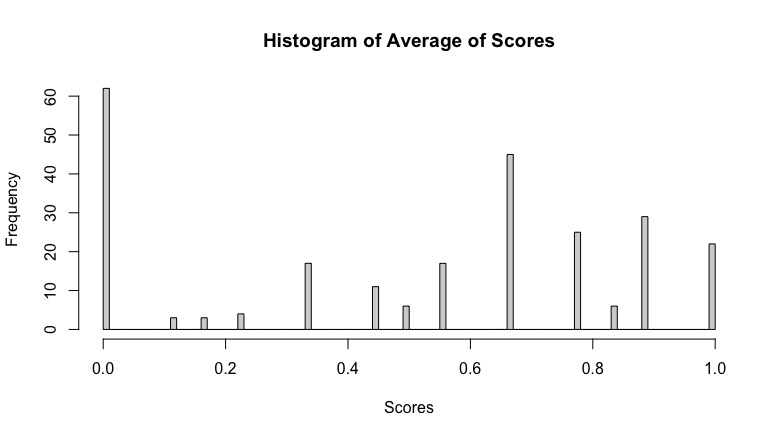
The histogram of "Average of Scores" looks like the following for 200 scores: