1
$\begingroup$

First time user here! I have some troubles in finding some statistic method that would fit my purpose for testing bivariate frequency table. Below is a sample frequency table I have created with ordinal data.

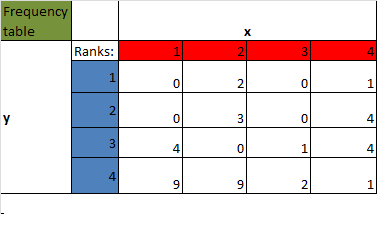
enter image description here

The rows and columns are labeled with ranks from 1 to 4. As you can see, most of my data is concentrated at the bottom-left corner for the sample. My goal is to quantitatively define this characteristic and perhaps perform a statistical test for estimating the dispersion in the population.

I have tried to the Chi-squared contingency table and Fisher's exact, but my table contains a few zeros cells.

Please advice any other appropriate test that I should attempt. Thank you.

ADDed: The experiment is designed as follow,

Four stocks are picked and their expected return and standard deviation are measured for 40 observations/periods. Within each observation, rankings are assigned to each stock for both y (expected return) and x (standard deviation) as 1 being the lowest and 4 being the highest. The frequency table above focuses on one of the four stocks.

if most of the observations fall into region of (y >= 3 & x <=2), I would characterize this stock as "low risk, high reward" option.

Improve this question
$\endgroup$
6
  • $\begingroup$ Please clarify your hypotheses/question of interest in detail $\endgroup$
    – Glen_b
    Commented Jul 5, 2014 at 1:15
  • $\begingroup$ Hi Glen, thank you for time. I have just added the details of my experiment to the original question. For this bivariate problem, I would like to test if the stock as shown above is "low risk, high reward" option (y >= 3 & x <= 2). I have attempted a hypothesis test using Wilcoxon signed rank test for the difference in rank. For instance, it failed to conclude there are significant difference in ranks for a sample that has 20 pairs of (1,4) and 20 pairs of (4,1).... does that make sense? Thank you again $\endgroup$
    – reckless pikachu
    Commented Jul 5, 2014 at 2:35
  • $\begingroup$ Please explain your question of interest in words, without any reference to ranks, names of tests or indeed any jargon at all (especially not statistical jargon). What are you trying to do? $\endgroup$
    – Glen_b
    Commented Jul 5, 2014 at 3:25
  • $\begingroup$ Given the return and risk profile of each stock for the 40 periods,I want to compare the stocks and see which one would offer my relatively higher return with lower risk. $\endgroup$
    – reckless pikachu
    Commented Jul 5, 2014 at 3:31
  • $\begingroup$ "See which one would offer higher return with lower risk" sounds like estimation, not hypothesis testing. (This may be why it was difficult for me to identify the hypotheses.) $\endgroup$
    – Glen_b
    Commented Jul 5, 2014 at 4:19

1 Answer 1

Reset to default
1
$\begingroup$

Because both the $\chi^{2}$ test and Fisher's exact test ignore the ordering of the categories in your variables, these are not appropriate tests. However, Spearman's r (sometimes Spearman's $\rho$) is a nonparametric statistic measuring monotonic association between two variables which can capture such ordering, such as $x$ and $y$. It is more or less Pearson's correlation coefficient applied to ranks. You can easily construct a $t$ test statistic to pose and answer the question "is the monotonic association statistically different than zero?" With a little more work, you can also pose and that question for a null hypothesis with a non-zero value.)

Constructing the correlation coefficient, $r_{\text{s}}$, is not terribly difficult:

$$r_{\text{s}}=1 - \frac{6\sum_{i=1}^{n}{d_{i}^{2}}}{n\left(n^{2}-1\right)}$$

Where $d_{i}$ is the difference in (independently) ranked values of $x$ and $y$.

Constructing a t test statistic with $n-1$ degrees of freedom for H$_{0}\text{: }\rho_{\text{s}} = 0$ is likewise not too difficult:

$$t = r_{\text{s}}\sqrt{\frac{n-2}{1-r_{\text{s}}^{2}}}$$

Improve this answer
$\endgroup$
3
  • $\begingroup$ Alexis, I appreciate your comment. But I would like to add that my goal isn't to test the correlation, but to find the dispersion of this bivariate frequency. For instance, is my data centered in one of the corners. For the ordinal data, I ran the wilcoxon test for examine the rank difference between x and y, but it doesn't produce a good result for me since it is testing the median. $\endgroup$
    – reckless pikachu
    Commented Jul 4, 2014 at 21:46
  • $\begingroup$ What precisely do you mean by "dispersion?" For example, do you mean something like variance? Because a t approximation to the variance is built into the test statistic I provided. Also: are your data paired? That wasn't clear from the question. $\endgroup$
    – Alexis
    Commented Jul 5, 2014 at 0:53
  • $\begingroup$ Hi again, I added more details to the original problems. Yes, my observations (40 of them) are pairs. Each observation has a rank in expected return and one in standard deviation ("see the added notes for more detail"). In summary, I want to test if most of data fall within certain region in my frequency table. $\endgroup$
    – reckless pikachu
    Commented Jul 5, 2014 at 2:38

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.