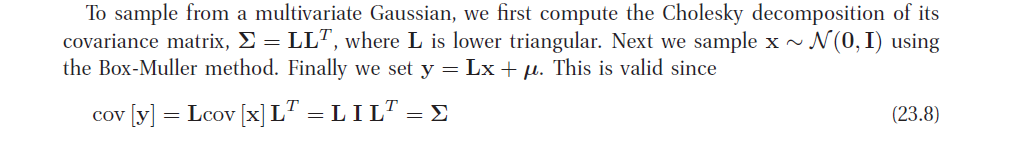1
$\begingroup$

i am reading "machine learning - a probabilistic perspective" by Kevin Murphy - who states the following in the chapter on monte carlo inference. i understand that cov[y] = $\Sigma$, but i do not see why this implies that $y=Lx+\mu$, and why this is equal to multivariate Gaussian sampling. Any hints much appreciated.

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

You basically reverse the logic. He plugged $y=Lx+\mu$ into covariance formula, and showed that if you do it then you'll get the given covariance matrix $\Sigma$. This means that sampling $x$ from independent standard normals will give you the required covariance matrix.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks. And $\mu$ drops out because it is a linear shift and does not affect the covariance? $\endgroup$
    – Wouter
    Commented Jul 26, 2015 at 19:49
  • $\begingroup$ @Wouter, yes, just like in a univariate case: $cov[ax+b]=a^2 cov[x]$ $\endgroup$
    – Aksakal
    Commented Jul 26, 2015 at 19:53

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.