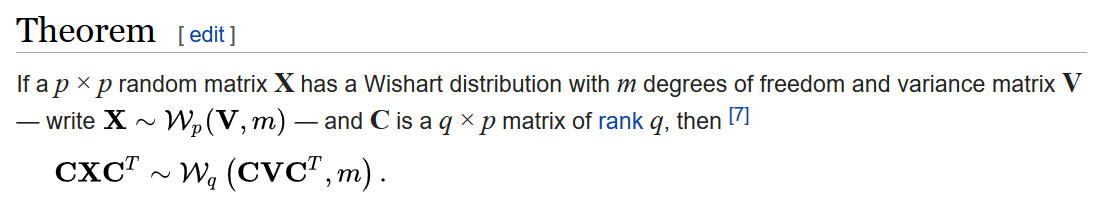5
$\begingroup$

If $X$ has a Wishart distribution $W_p(n,\Sigma)$ , what's the distribution for $cX$ where $c>0$ ?

I know that for a $\chi^2_n (x)$ distribution with $n$ degrees of freedom, $c\chi^2 $ follows $\Gamma(x;\frac{n}{2},2c)$ for the scaling property of gamma distribution so the answer should not be so obvious.

This question arose because $\frac{1}{n} W$ should be the the correct distribution for the sample covariance, according to what I understand from theory.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

I think I just found an answer but I leave open the question for the sake of the community:

Actually using the theorem found here (1) : $$CXC^t \sim W(n,C\Sigma C^t) $$ and taking $C$ as the diagonal matrix : $$\left( \begin{matrix} \sqrt{c} & \ldots & 0 \\ \vdots & \ddots & \vdots \\ 0 & \cdots & \sqrt{c} \\ \end{matrix} \right) $$

Ergo in this case $cX$ should be $W(n,c\Sigma)$ distributed. Relating to my example for the sample covariance, $C = \text{diag}(\frac{1}{\sqrt{n}})$. Hence, $\frac{1}{n}X=\hat{\Sigma}$ should be simply $W(n, \frac{1}{n}\Sigma) $ distributed.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ You've changed your notation in midstream from $c$ to $n$, it appears. You asked about $cX$, but you are responding with $1/n$... and since $\Sigma$ is a parameter, it doesn't have a distribution (referring to your last sentence.) $\endgroup$
    – jbowman
    Commented Mar 10, 2018 at 17:48
  • $\begingroup$ Yes , i know. The question arose for the purpose of covariance sample matrix distrubution, where I have $ c=\frac{1}{n} $ . It's a dummy scalar variable. Of course in this case $X=n\hat{\Sigma}$ has a distribution. I edited the last $\Sigma$ with an hat. $\endgroup$
    – omega
    Commented Mar 10, 2018 at 17:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.