1
$\begingroup$

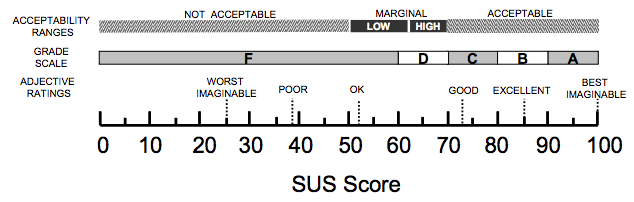
Imagine you have a set of scores between 0 and 100 ("SUS Scores"). Based on the distribution of hundreds of these scores people have tried to provide adjective "grades" for these scores such as "ok", "aweful", "good", ... This distribution is not centered around 50 and these grades do not necessarily have the same range of scores.

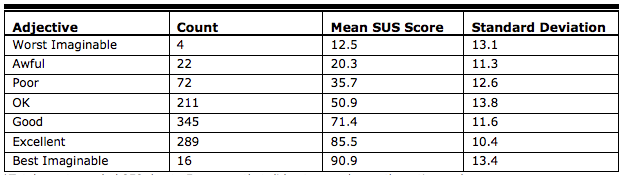
However, in the paper the categories are defined as a set of means with standard deviations. No clear boundaries are provided: SUS Score Categories

I would like to derive concrete thresholds / intervals so I can for instance say "if the score is between 55 and 68, the grade is 'good'". Is this possible with the given data?

The paper provides the following "intervals" but I have no idea how they relate to the means provided earlier. I have also noticed other sources have attempted the same and even came to different conclusions.

SUS Intervals

I have tried plotting normal curves around the provided mean with the provided standard deviations, but this has not provided me with any insight. Can anyone help me to estimate the intervals for these adjective grades?

My question is based on this paper, but the background info is not actually important to the question: http://uxpajournal.org/determining-what-individual-sus-scores-mean-adding-an-adjective-rating-scale/

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

Without some additional assumptions, you cannot do this. However, the scores look roughly (OK, very roughly) normal (across all categories) so you can compute the overall mean and sd and look at the distribution of a normally distributed sample with the size of the one you have. That won't give you an exact answer, but it should give a guide. If you generate a bunch of samples, it might help.

Note that if the overall distribution is normal, the subgroups will not be (e.g. the left tail of a normal is not remotely normal).

Improve this answer
$\endgroup$
2
  • $\begingroup$ What "additional assumptions" would be needed to succeed? What would a minimal set of such assumptions be? Would any of them have to be stronger than assuming the data are a random sample of a population--which is a default implicit assumption in such questions? $\endgroup$
    – whuber ♦
    Commented Jan 27, 2019 at 14:15
  • 1
    $\begingroup$ You'd have to make some assumptions about the distribution. $\endgroup$
    – Peter Flom
    Commented Jan 27, 2019 at 15:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.