5-year EFS > 5-year OS

Question

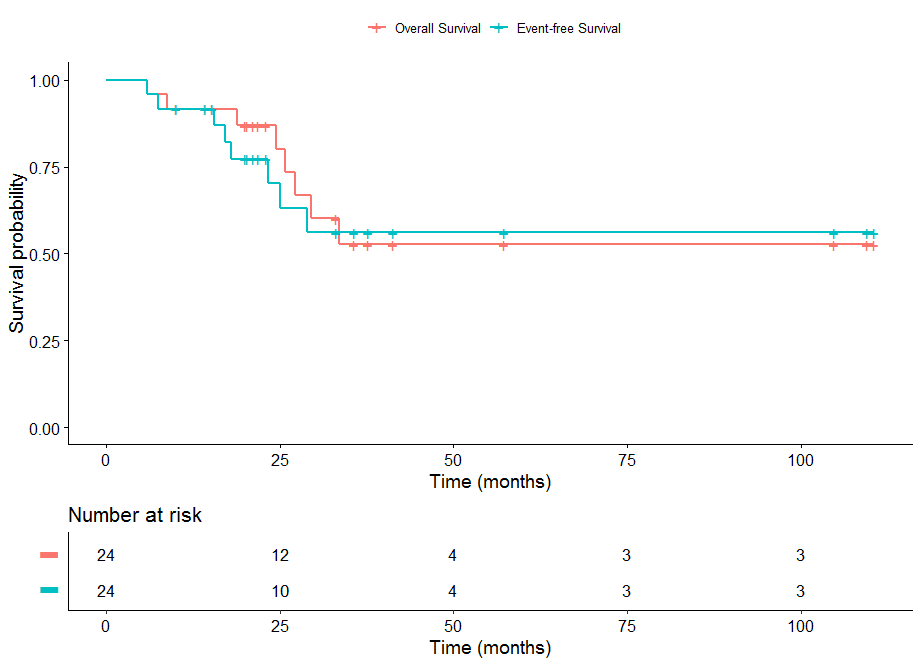
I had a data of 24 cancer patients; their 5-year overall survival (i.e. event as death) was around 57%, however, when I calculated 5-year event-free survival for the same cohort, defining the event as death or relapse, I got 61%.

I have scrutinized the data and I am 99.99% sure there is nothing wrong with it, and I could totally see how this could result from the survival function. I thought Cross Validated community might give more meaningful insights.

Is there anything wrong with this? Did that happen to you before? If so, how do you report it?

Of notice, this didn't happen anymore when the follow up was updated and 2 more cases were included. I also noticed that all the cases that died had also experienced an event before death.

Any thoughts?

Answer 1

The pattern of events and censoring over time accounts for this small discrepancy. Remember that the size of a step down in a Kaplan-Meier curve, at each event, is based on the previous survival fraction and the number still at risk at the time of the event.

In your data there are 5 censorings in both curves at around 20-25 months. That's more than 20% of your initial cohort lost to follow up over a short period of time. These censorings occur after the 5th event in the event-free survival curve, but after only the 3rd death. The sizes of the 4th and 5th steps down in the overall-survival curve (with only 13 resp. 12 at risk by my count) are thus larger than the 4th and 5th steps down in the event-free survival curve (with 18 resp. 17 at risk), taking the overall survival curve to a lower survival fraction than the event-free survival curve after the same number of events. The pattern of lower overall survival than event-free survival after the same number of events continues thereafter. Once the number of events has reached 8 in both curves, the overall survival curve remains a bit lower.

As the most useful information in a survival curve is provided by the events, you might think about this as those 5 particular censorings having lost more information about overall survival (5 censorings after only 3 events) than about event-free survival (5 censorings after 5 events). In any case, the discrepancy is minor: a 4 percentage-point discrepancy with only 24 cases to start is pretty much just +/- one case.