I am trying to determine required number of days required to reach a significant result for a one-tailed independent sample test. I have sales data by day for my test and control group. The results per day are as follows:
My question: How do I interpret this number (91) exactly? 91 days needed for the test? Any help would be very much appreciated!
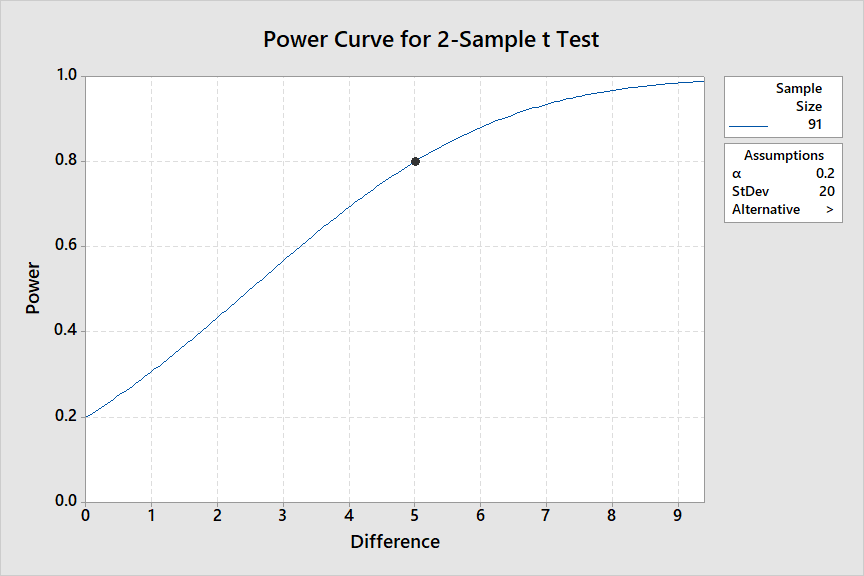
Apparently, you are doing a two-sample pooled t test of $H_0: \mu_1 = \mu_2$ vs $H_a: \mu_1 > \mu_2$ at the 20% level of significance and wishing to detect a difference of $\delta = 5$ thousand with power 80%, where the standard deviations of the two independent groups are each $\sigma = 20$ thousand. You found that a sample size if $n = 91$ is required.
Here is output from Minitab statistical software confirming this result:
Power and Sample Size
2-Sample t Test
Testing mean 1 = mean 2 (versus >)
Calculating power for mean 1 = mean 2 + difference
α = 0.2
Assumed standard deviation = 20
Sample Target
Difference Size Power Actual Power
5 91 0.8 0.800402
The sample size is for each group.
As this output says, you would need 91 days in each group (91 in the treatment group and 91 in the control group, for a total of 182 days of observations).
Notes: (1) The ratio $\delta/\sigma = 5/20 = 0.25$ is crucial to this computation.
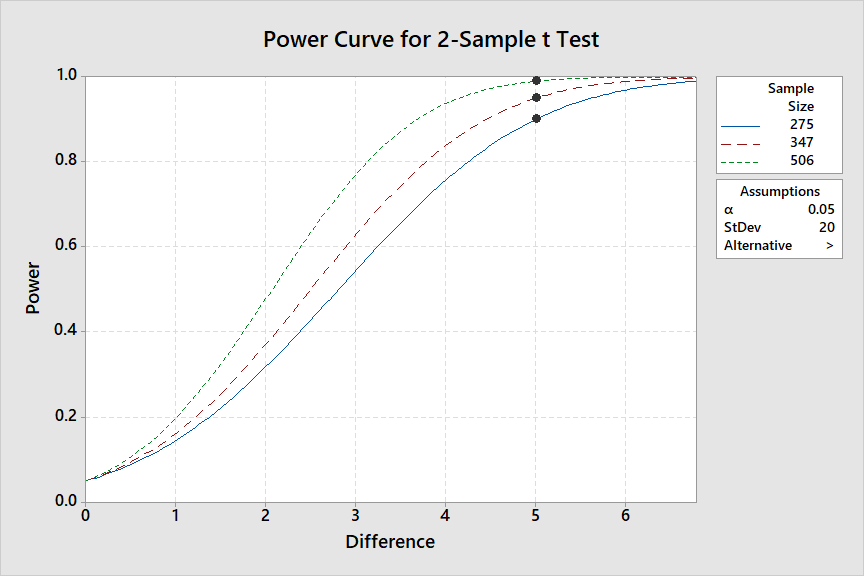
(2) The desired error probabilities are also crucial: if you want 5% and 95%, then the sample size required in each group increases to $n=347.$
Power and Sample Size
2-Sample t Test
Testing mean 1 = mean 2 (versus >)
Calculating power for mean 1 = mean 2 + difference
α = 0.05
Assumed standard deviation = 20
Sample Target
Difference Size Power Actual Power
5 275 0.90 0.900260
5 347 0.95 0.950006
5 506 0.99 0.990070
The sample size is for each group.
