Factor Analysis uses a single factor or multiple?

Question

I am not able to follow the explanation in the book 'Applied multivariate statistical analysis':

First, they nicely explain:

Suppose variables can be grouped by their correlations. That is, suppose all variables within a particular group are highly correlated among themselves, but have relatively small correlations with variables in a different group.Then it is conceivable that each group of variables represents a single underlying construct, or factor, that is responsible for the observed correlations. For example, correlations from the group of test scores in classics, French, English, mathematics, and music collected by Spearman suggested an underlying “intelligence” factor.

The paragraph nicely explains the basics, and it clarifies that each group represents a SINGLE factor.

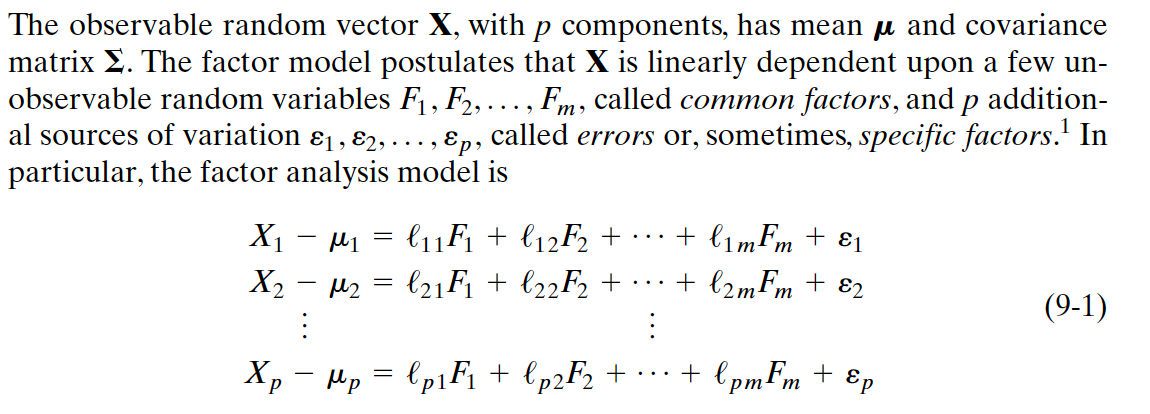
But then it contradicts in the formula:

(The formula specifies more than one factor, why?)

Following the previous analogy, in the formula... $X_1$ would be 'classics', $X_2$'french', $X_3$'english', $X_4$'math' and so on... then $F_1$ would be 'intelligence'.

Why in the introduction they say 'single' factor, and then the formula has multiple?

Furthermore, the expected value of the factor is assumed to be 0... why is that?

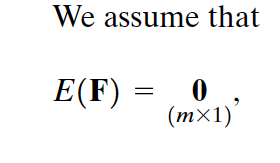
(this would mean that the expected intelligence is '0'?!)

Answer 1

That is, suppose all variables within a particular group are highly correlated among themselves, but have relatively small correlations with variables in a different group.Then it is conceivable that each group of variables represents a single underlying construct, or factor, that is responsible for the observed correlations.

You've missed an important part of the introduction, and then the example they give is confusing. In the text, they are saying that there are multiple groups of variables, and within each group the correlations are high with each other, but not so high with variables in other groups.

In the example (Spearman's research), they are talking about a famous argument about whether there is one general intelligence factor (usually called g). So, one outcome of a factor analysis could be that there is only one factor, but that isn't always the case.

Suppose I give a group of students a math test, a computer programming test, a physics test, an English test, a hands-on autoshop test, a test of manual dexterity, a practical tests of electrical repaid and plumbing repair. One hypothesis about this might be that the first 4 tests would correlate highly with each other, and less highly with the last 4, while the last 4 would correlate highly with each other and less highly with the first 4. So, we could have 2 factors in this case.

The number of factors you have is one of the more difficult problems with applying factor analysis.