1
$\begingroup$

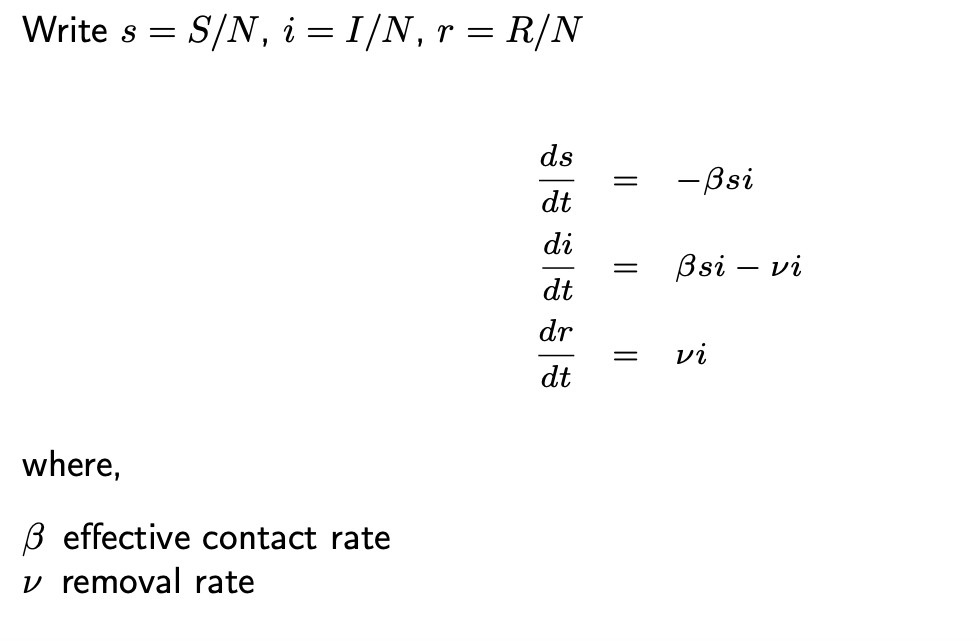
I was having trouble trying to understand the parameters of the simplest SIR model.

enter image description here

If beta is the effective contact rate and s is the percentage of people who are susceptible, then how do the units cancel out such that ds/dt is measured in percentage per unit time? When I multiply -ßsi out, the units cancel out to people/time, which isn't how ds/dt is measured. Am I missing something here?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

The variables $s, i, n$ are dimensionless. (You consider the dimension 'percentage' but that is not a dimension. A percentage is dimensionless.)

So the differential on the left $\frac{di}{dt}$ has units 'per unit time' and not 'percentage per unit time'.

On the right the term $\beta si$ has also units 'per unit time'. The $\beta$, a rate, has units 'per unit time', and the $s$ and $i$ are dimensionless.

Improve this answer
$\endgroup$
6
  • $\begingroup$ thanks for keep answering SIR model related questions. I have been thinking an interesting problem: if I do not know SIR model, just use the old machine learning and curve fitting thing for number of infections over time, I may get some polynomial fit on I(t). What is pros and cons for polynomial fit and SIR fit? I may want to ask such question later in CV. $\endgroup$
    – Haitao Du
    Commented Apr 21, 2020 at 9:46
  • $\begingroup$ @HaitaoDu That may certainly be an interesting question on its own. I do not have much knowledge of machine learning, and despite some understanding of fitting with regularization, I am still skeptical about the fits with neural networks (machine learning is a bit ambiguous and I am throwing in NN, I am not sure what you refer to). I believe that these sort of technologies only work in the proper invironment.... $\endgroup$
    – Sextus Empiricus
    Commented Apr 21, 2020 at 10:03
  • $\begingroup$ ... There is apparently some machine learning type model and it is being criticized by the theoretical, ab-initio-oriented people, for not providing any insight. Machine learning may certainly provide predictions (as good as they can get which, I believe, is not much), but it will not provide much insight. I believe that we are operating with limited knowledge/data, but still can get some of the principles. $\endgroup$
    – Sextus Empiricus
    Commented Apr 21, 2020 at 10:13
  • $\begingroup$ .... normally I would be less skeptical about machine learning, but with the covid-19 data, I suspect a lot of bias in the data and also I am very uncertain about the pattern that a machine learning method would learn. There is not a lot clear and unbiased data and the learning will be likely a lot biased. $\endgroup$
    – Sextus Empiricus
    Commented Apr 21, 2020 at 10:18
  • $\begingroup$ I think that problem can be viewed as parametric or non-parametric model, or similar to generative model vs. discriminative model, the key idea is that, if the model assumption is right, then parametric (SIR) model will be better. On the other hand, if SIR is way off, then non-parametric will be better. $\endgroup$
    – Haitao Du
    Commented Apr 21, 2020 at 10:22

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.