0
$\begingroup$

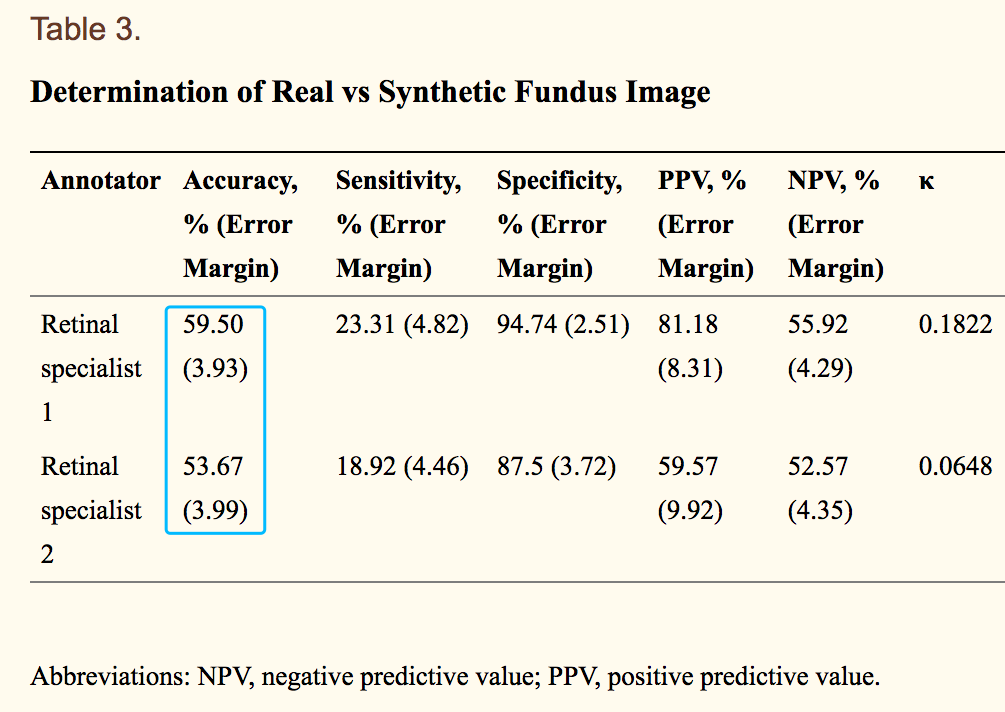
A paper Assessment of Deep Generative Models for High-Resolution Synthetic Retinal Image Generation of Age-Related Macular Degeneration performed an experiment and the result is

Retinal specialists could not distinguish real from synthetic images, with an accuracy of 59.50% (error margin, 3.93%) for retinal specialist 1 and 53.67%(error margin, 3.99%) for retinal specialist 2.

I partly understand what the researchers did, they invited 2 retinal specialists do something like the following respectively,

They gave specialist 1 a set of images, told they that set consists of real images and synthetic images (simply, some kind of fake images), asked they pick real images from the set, and stat how many "real images" in the ones (let's call it #specialist_1_picked) specialist 1 picked are actually "real images" (let's call it #actually_real_in_1)

They asked specialist 2 do the experiment separately and stated the same metrics.

enter image description here

The question is what is the exact formula to compute those accuracies 59.50% and 53.67%. Could someone please give a hint? Thanks in advance.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

Accuracy (as well as the other abbreviations in your table) is defined on the Wikipedia page on sensitivity and specificity. Specifically, accuracy is defined as

$$ \text{ACC}:=\frac{\text{TP}+\text{TN}}{\text{P}+\text{N}}. $$

Accuracy is not a good measure for assessing classification models.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thank you. Is the #actually_real_in_1 in my OP the TP in your formula? $\endgroup$
    – WXJ96163
    Commented Apr 6, 2020 at 14:44
  • $\begingroup$ Yes, that's it. $\endgroup$
    – Stephan Kolassa
    Commented Apr 6, 2020 at 14:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.