1
$\begingroup$

In a previous posting on this site RepulsiveBehavior I attempted to detail a quantum-information-theoretic separability/entanglement problem I am pursuing. Detailed issues of sampling sizes for a data set being developing were raised and perhaps obscured the chief question I hope to have addressed/clarified.

That is, based on my study BlochRadiiRepulsion, I believe that I am dealing with a symmetric copula $f(x,y)=f(y,x)$ defined over the unit square for which the values of $f(x,x)$ assume relative minima.

Might there be any standard copulas (at least for certain parameter settings) exhibiting such behavior that I could try to fit to these data being developing? My attempts to do so MathematicaQuestion have not so far been successful in this regard.

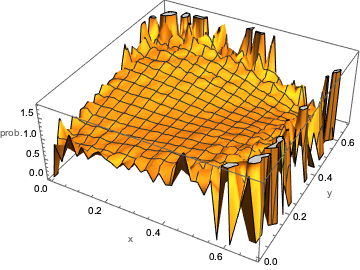
Here is a plot of the pdf based on my sampling procedure

CopulaPDF

The relative minima along $x=y$ are discernible.

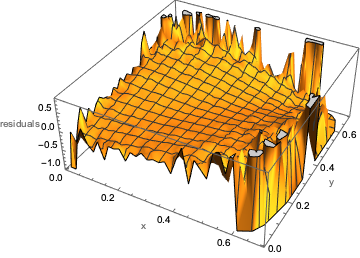
Here is a further plot of the residuals from this pdf of a weighted least-squares fit of the Ali-Mikhail-Haq (AMH) model with estimated parameter 0.0192858.

ResidualsFromAMHfit

Improve this question
$\endgroup$
7
  • $\begingroup$ By relative minima do you mean the density along the $y=x$ axis is the lowest within the unit square? $\endgroup$
    – Richard Hardy
    Commented Dec 30, 2021 at 12:19
  • $\begingroup$ Thanks for the comment, RH! Subject to my sampling and obvious lack of full knowledge of the governing process (for which I am searching for possible rules), it appears that if one deviates from the x = y line, the probabilities increase. $\endgroup$
    – Paul B. Slater
    Commented Dec 30, 2021 at 13:47
  • $\begingroup$ If you consider the relationship between the copula and the conditional distributions, you will immediately deduce that the copula is non-decreasing in both arguments. Consequently, the only relative (strict) minimum it can possibly have is at $(0,0).$ $\endgroup$
    – whuber ♦
    Commented Dec 30, 2021 at 15:55
  • $\begingroup$ whuber--does this comment extend as well to the pdf, my principal focus of analysis? In any case, I'm interested in models, in which strong repulsion (relatively low values) is shown for x = y, if still not over the entire line. $\endgroup$
    – Paul B. Slater
    Commented Dec 30, 2021 at 16:14
  • 1
    $\begingroup$ The illustration helps. It makes one wonder: why do you even want to use a copula? What is it intended to accomplish? Instead of focusing on a specific method, you are likely to get more useful answers by articulating your goals (along with descriptions of the information you have to work with and your constraints, of course). That would allow people to suggest methods that might be better than the one you are focusing on. $\endgroup$
    – whuber ♦
    Commented Dec 31, 2021 at 16:11

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.