0
$\begingroup$

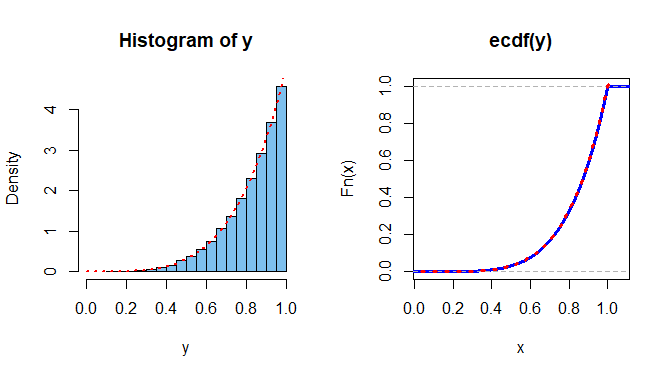
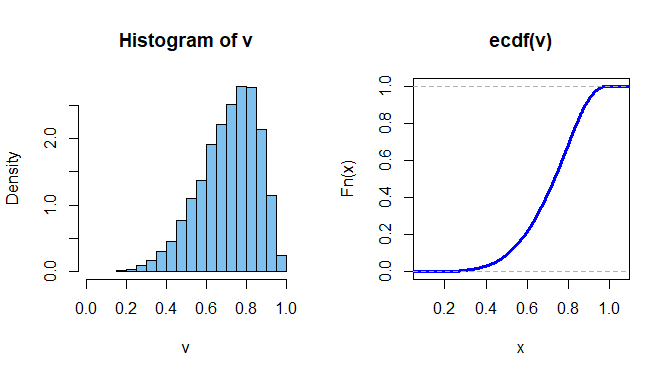
𝑓(𝑦) = 5𝑦^4; 0 ≤ 𝑦 ≤ 1 A group of 3 friends order small cups of soda, from the soda dispenser. If the 3 small cups are considered a random sample from the dispenser fills, find the probability distribution for the minimum fill and answer, according to the customer satisfaction benchmark. If customer satisfaction is benchmarked as satisfied by a fill of 2/3 or more for their chosen cup size, what is the probability, to 3 decimal places, that all three friends are satisfied with their small cup? (You must answer in terms of the minimum order statistic 𝑌1′.)

Using order statistic, I can find the f(Ymin) with the equation: n*[1-F(y)]^(n-1)*f(y), where F(y) is the CDF and f(y) is the given pdf from above (1st line). I can find the probability of f(Y'1) or f(Ymin) by taking the integral of the pdf. But to find all three friends are satisfied, would I then cube the probability that I found since all three friends success are independent?

Improve this question
$\endgroup$
3
  • $\begingroup$ Welcome to the site! Please take the tour to learn how to ask good questions here. Your explanation of what you have done so far is welcome, but it is partial and a bit sketchy. Explicitly, what is the CDF of $Y?$ What answer do you get for the CDF of the minimum $V$ of three? How do you use the CDF of $Y$ to get the probability $P(Y \ge 2/3)$ that the a given one of the friends is satisfied? Is that related to $P(V \ge 2/3)?$ $\endgroup$
    – BruceET
    Commented Mar 13, 2022 at 17:29
  • $\begingroup$ I would assume in the equation for finding joint pdf: fy1',y2'(u,v) we would substitute u for y in fy1' and v for y in fy2'. Here fy1' = 15(y^15+2y^9+y^4) and fy2'=30y^9-30y^15 and CDF of y2' Fy2'(y) = 3y^10-2y^15. So we can the double integrate with respect to dv and du. We do the integral of: 6*(1-Fy2'(v))*fy1'(u)*fY2'(v) dv du Next, I am struggling with finding what the limits of the integration would be? $\endgroup$
    – confusedaboutstats
    Commented Mar 13, 2022 at 18:28
  • $\begingroup$ You need to be more careful using JaX for math notation, your equations are difficult to decipher. Perhaps you are confusing even yourself. // Carefully, state the support of each distribution used. All random variables $(Y$s and $V)$ have the unit interval as support. $\endgroup$
    – BruceET
    Commented Mar 13, 2022 at 21:12

1 Answer 1

Reset to default
0
$\begingroup$

The distribution of $Y$ is a member of the beta family of distributions: $Y \sim \mathsf{Beta}(5, 1),$ with density $f_Y(y) = 5y^4,$ for $0 \le y \le 1,$ (otherwise $0);$ mean $\mu = \int_0^1 x(5y^4)\,dy = 5/6;$ and CDF $F_Y(y) = \int_0^1 5t^4\, dt = y^5$ for $0 \le y \le 1$ $(0$ for $y\le 0; 1$ for $y \ge 1.$

Here is a simulation of $100\,000$ realizations of $Y$ which match the PDF and CDF shown above (plotted in red below):

set.seed(2022)
y = rbeta(10^5, 5, 1)
par(mfrow=c(1,2))
hist(y, prob=T, xlim=0:1, col="skyblue2")
 curve(5*x^4, add=T, col="red", lwd=2, lty="dotted")
plot(ecdf(y),lwd=3, col="blue")
 curve(x^5, add=T, col="red", lwd=3, lty="dotted")
par(mfrow=c(1,1))
summary(y)
   Min. 1st Qu.  Median    Mean 3rd Qu.    Max. 
 0.1084  0.7579  0.8717  0.8341  0.9448  1.0000

enter image description here

Here is a similar simulation of $V = \min(Y_1,Y_2,Y_3),$ where the $Y_i$ are independent and distributed as above. With $100\,000$ simulated values of $V,$ sample summaries should agree with population summaries to about two decimal places.

set.seed(314)
v = replicate(10^4, min(rbeta(3,5,1)))
par(mfrow=c(1,2))
 hist(v, prob=T, xlim=0:1, col="skyblue2")
 plot(ecdf(v),lwd=3, col="blue")
par(mfrow=c(1,1))
summary(v)
   Min. 1st Qu.  Median    Mean 3rd Qu.    Max. 
 0.1548  0.6200  0.7318  0.7112  0.8204  0.9899 
mean(v > 2/3)
[1] 0.6547

enter image description here

I will let you find an analytic formula for the CDF of $V.$ It may be helpful to notice that $$P(V > v) = P(Y_1 > v, Y_2 > v, Y_3 > v),$$ for $0 \le v \le 1.$

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.