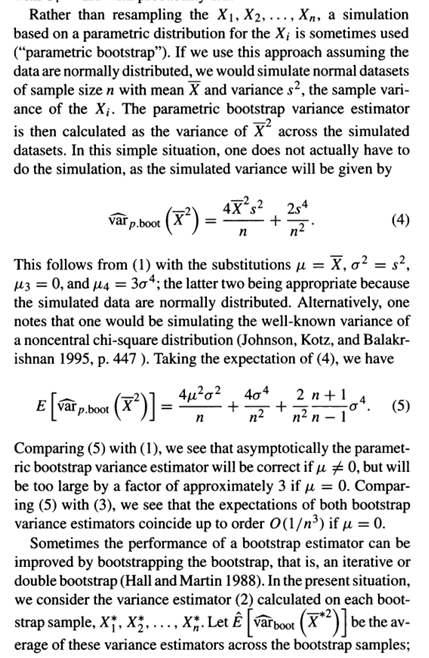
I'm quite unclear how they derived (5) from (4). Since $\bar{X}$ and $s^2$ are unbiased estimators, I believe my lack of follow through comes from not knowing how to compute $\mathbb{E}[\bar{X}^2 s^2]$. Any help is appreciated.
1 Answer
$\bullet$ Since the data is normally distributed, $\bar X$ and $X_i-\bar X$ and thus $s^2$ are independently distributed.
$\bullet$ The variance of $s^2$ is
$$\operatorname{Var}[s^2] = \frac{2\sigma^4}{(n-1) }.\tag 1\label 1$$
Now
\begin{align}\frac{4}n \mathbb E\left[\bar X^2s^2\right]+ \frac{2}{n^2}\mathbb E\left[s^4\right]&=\frac{4}n \mathbb E\left[\bar X^2\right]\mathbb E\left[s^2\right]+ \frac{2}{n^2}\mathbb E\left[s^4\right]\\ &=\frac{4}n \mathbb E\left[\operatorname{Var}\left[\bar X\right]+ \left(\mathbb E[\bar X]\right)^2\right]\mathbb E\left[s^2\right]+ \frac{2}{n^2}\mathbb E\left[\operatorname{Var}\left[s^2\right]+ \left(\mathbb E\left[s^2\right]\right)^2\right] \\ &= \frac{4}n \left[\frac{\sigma^2}n +\mu^2\right]\sigma^2+ \frac{2}{n^2}\left[\frac{2\sigma^4}{n-1}+\sigma^4\right]~~\textrm{using}~~\eqref{1}\\ &= \frac{4\sigma^4}{n^2} +\frac{4\mu^2\sigma^2}{n}+ \frac{2}{n^2}\left[\frac{2\sigma^4+n\sigma^4-\sigma^4}{n-1}\right]\\ &= \frac{4\sigma^4}{n^2} +\frac{4\mu^2\sigma^2}{n}+ \frac{2}{n^2}\frac{(n+1)\sigma^4}{n-1}.\end{align}