7
$\begingroup$

This is a follow-up to my previous question: "Basic understanding of control variables in observational studies"

I have understood the answer, but am still trying to figure out what I think of the Wikipedia example that I'd cited.

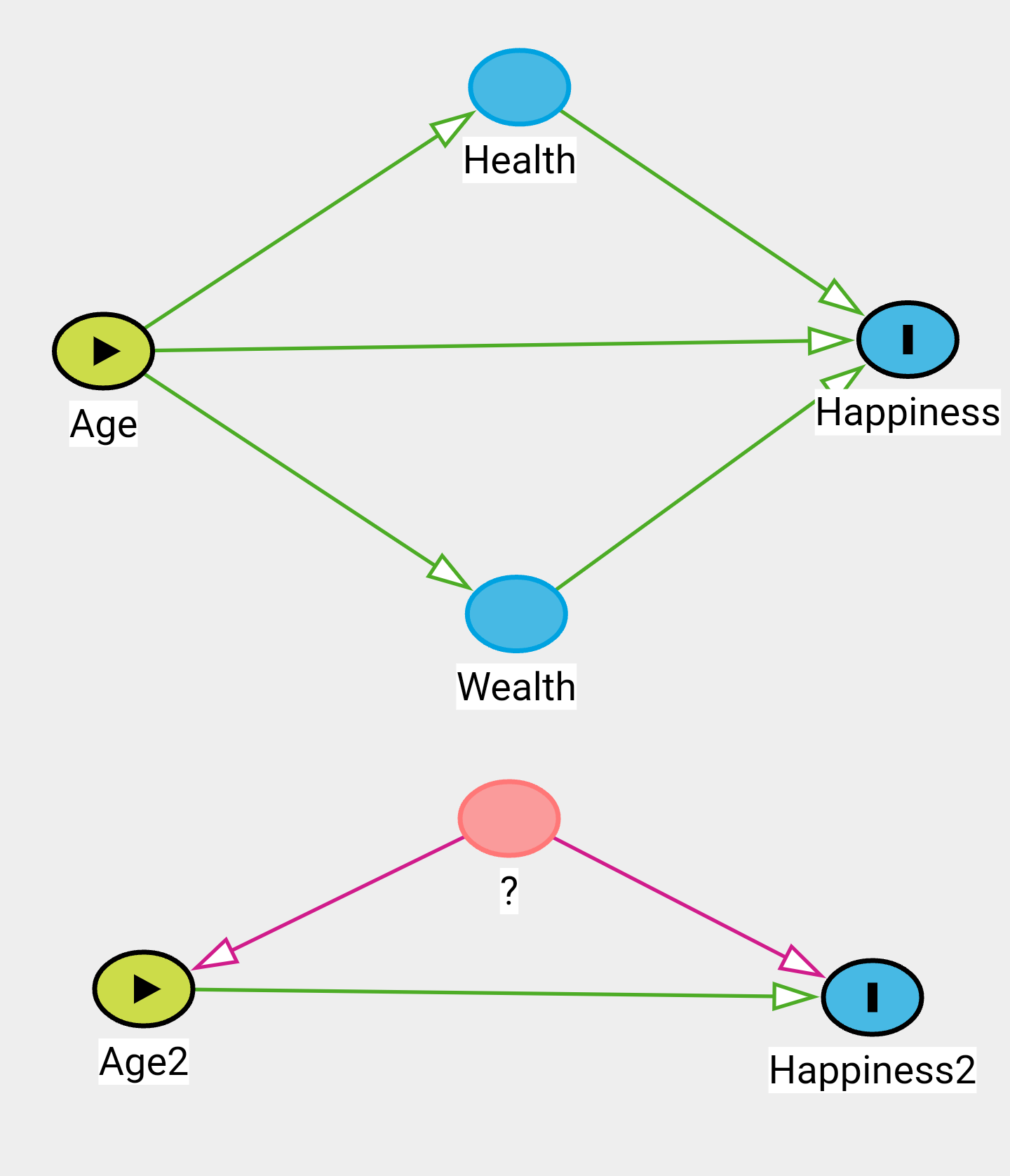
Basically, the example is the effect of age on happiness, and discusses whether you should ever control for health. The example says no, because:

To identify the control variables needed here, one could ask what other variables determine not only someone's life satisfaction but also their age. Many other variables determine life satisfaction. But no other variable determines how old someone is (as long as they remain alive). (All people keep getting older, at the same rate, no matter what their other characteristics.) So, no control variables are needed here.

What I understand by this explanation is that age can never (or close to never) be confounded if it is the independent variable in the study.

I have three questions about this explanation:

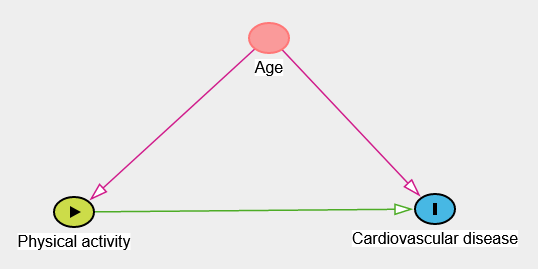
  1. Is it not mixing up the individual with the population? E.g., let's say wealth completely determines happiness and has a strong effect on longevity. You have a population of 100 people, 60 are age sixty and 40 age seventy. Happiness is measured as 1 = happy and 0 = not. At sixty, half the people are wealthy. At seventy, 3/4s are. Average happiness at sixty = 0.5 (30x1/60) and at seventy = 0.75. Happiness correlates positively with age, but controlling for wealth eliminates this. In this case, shouldn't one control for wealth?

  2. Is their a clear theoretical reason to, or not to, control for health in the Wiki example? To my understanding, if Age = X, Happiness = Y, and Health = Z, you have a number of valid relationships: X → Y, X → Z → Y, Z → X, and U → Z. This seems to give contradictory reasons to, or not to, control.

  3. Does it depend on what question you want to ask? In my original question, FP0 said:

Maybe they consider that since age changes by itself without external influence, then they want to consider that the additional effects of the variables I imagined above should be "included" in the global effect of age, since age has a causal impact on them. In my opinion it depends on whether you want to aggregate the different effects of age since age is causal on those factors, or if you want to identify them individually, and estimate the remaining effects of age separately.

If the answers to 1 and 3 are 'yes,' I have issues with the Wiki example.

Improve this question
$\endgroup$
2
  • $\begingroup$ You can substantially edit your title if needed without mentioning [fixed title]. $\endgroup$
    – User1865345
    Commented Dec 26, 2022 at 12:11
  • 1
    $\begingroup$ I think the qualifier "(as long as they remain alive)" is really important here. $\endgroup$
    – Joseph Sible-Reinstate Monica
    Commented Dec 26, 2022 at 23:10

2 Answers 2

Reset to default
10
$\begingroup$

First image below: With variables 'health' and 'wealth' you describe a situation with mediators. Age has a causal effect on health and wealth, and these in their turn have an effect on happiness.

Second image below: Controlling would relate to a (confounding) variable that has a causal effect on both the exposure variable (age in the example) and the outcome variable (happiness). Since there are few parameters that cause age, there is not much to control for.

example

I agree that the example is not so strong and in the example this is also nuanced by the sentence:

(as long as they remain alive)

There might be a confounding survivorship bias if one would argue that age causes happiness because we observe older people are more happy. The bias is that one assumes that there is no causal relationship from happiness to age which might be false.

Improve this answer
$\endgroup$
13
  • 1
    $\begingroup$ Your title seems to be a different question compared to the situation/example in your body text "Should you ever control for the independent variable 'age' in an observational study?" Here one studies the effect of some variable $X$ on another variable $Y$ and 'age' can be a confounding variable in that relationship by having causal relationships with $X$ and $Y$. $\endgroup$
    – Sextus Empiricus
    Commented Dec 25, 2022 at 21:01
  • $\begingroup$ The reason for the title is because the Wiki example that I cite maintains (if I read it correctly) that you should never control for the ind var age because it cannot be affected. That is my 'top level' question. $\endgroup$
    – sean.mcgrath
    Commented Dec 25, 2022 at 21:09
  • $\begingroup$ Re. the first image: yes, this I believe that I understand this. If health or wealth is a mediator, it should not be controlled. My Q1 is related to my title. I give an example to challenge that one can never control for age. Assuming that the answer to Q1 is that age can be determined (e.g., by age if you are talking about the general population), my Q2 is whether health can then be seen as a common cause of both age and happiness. $\endgroup$
    – sean.mcgrath
    Commented Dec 25, 2022 at 21:18
  • $\begingroup$ @sean.mcgrath the phrase 'control for age' relates to the situation where age is the confounding variable in another relationship. You control for age when you perform an experiment where you keep 'age' constant or when you correct for it in the analysis. The example on the Wikipedia page is the situation when 'age' is a variable that is being confounded (and they argue that that situation doesn't often occur). $\endgroup$
    – Sextus Empiricus
    Commented Dec 25, 2022 at 21:42
  • $\begingroup$ I see. It looks like I misused the terminology. I struggled a bit on the terminology because the Wiki example only wrote about 'control.' Should my title then read "Can age ever be confounded if it is the independent variable in an observational study?" As far as I can see, they argue that it can never occur. Is this incorrect? $\endgroup$
    – sean.mcgrath
    Commented Dec 25, 2022 at 21:58
6
$\begingroup$

Observational studies adjust for age all the time! I would not be surprised if age was the most common adjustment variable in observational studies.

If age is the exposure (as in both examples of @SextusEmpiricus), the variable of interest, then you must include it in the model (how else are you going to estimate its effect?) and it - by definition - cannot be a confounding variable and be "adjusted for". Adjusting for just means that you include the variable in some model or condition on it in some other way (e.g. stratified analysis).

You would adjust for age if it isn't the variable of interest and lies on a backdoor path between your exposure and outcome in the DAG. An extremely simple example is shown below:

DAG

Here, we're interested in the causal effect of physical activity (PA) on cardiovascular disease (CVD). If age is both causally related to physical activity and cardiovascular disease, there is a backdoor path from PA <- Age -> CVD. By adjusting for age (conditioning on it), you block the path. So in this example, you must adjust for age to get the unbiased causal effect of PA on CVD.

Improve this answer
$\endgroup$
1
  • $\begingroup$ it looks like my terminology was incorrect. I am interested in the case where age is the independent variable. My first question, if I understand the wording correctly, is whether in this situation 'age' can possibly be confounded (it seems the Wiki page says that it cannot). $\endgroup$
    – sean.mcgrath
    Commented Dec 25, 2022 at 22:00

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.