Interpretation of a definition related to stochastic processes

Question

Here is some context.

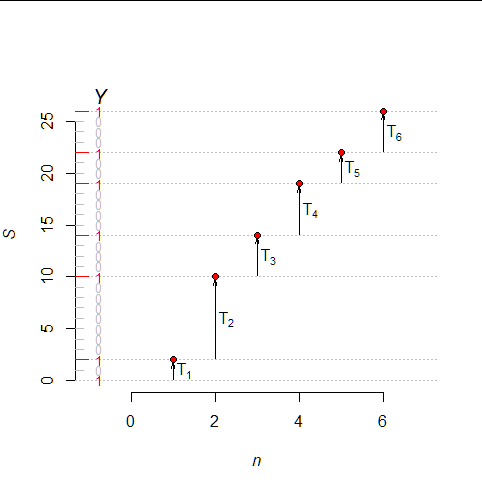
Let $(T_{n})_{n\geq 1}$ be an i.i.d. sequence of random variables taking values in $\{1,2,\ldots\}\cup\{\infty\}$ with common distribution $(q_{k})_{k\geq 1}$. We call discrete (undelayed) renewal process the process $(Y_{n})_{n\geq 1}$ defined through $Y_{0} = 1$ and, for any $n\geq 1$, \begin{align*} Y_{n} = \textbf{1}\{T_{1} + T_{2} + \ldots + T_{i} = n \ \text{for some} \ i\} \end{align*}

I am concerned about the following claim:

Observe that $T_{n}$ is the distance between the $(n - 1)^{\text{th}}$ and the $n^{\text{th}}$ occurrence of 1 in $(Y_{n})_{n\geq 1}$.

Could anyone help me in understanding the last assertion?

Answer 1

Let $S_1=T_1$ and, for all $i\ge 1,$ let $S_{i+1} = T_{i+1} + T_i$ be the sequence of cumulative sums. $Y_n$ is the indicator that the graph of $S,$ $\{(i, S_i)\mid i = 0, 1, 2, \ldots \},$ attains an elevation of $n$ at some point.

The vector $(Y_n)_{\,n\ge 1}$ therefore is a sequence of zeros and ones with a one in position $n$ precisely when $n$ appears in the graph of $S.$ Because $T_i \gt 0$ for all $i,$ the sequence $S$ is strictly increasing, whence the ones in $Y_n$ appear at the positions $S_1 \lt S_2 \lt S_3 \lt \cdots$ $\lt S_{n-1} \lt S_n \lt \cdots.$ The $n-1^\text{st}$ and $n^{\text{th}}$ occurrences of $1$ are at positions $S_{n-1}$ and $S_n,$ a distance $S_n - S_{n-1} = T_n$ apart, QED.

---

$Y$ is a point process on the natural numbers. The $T_n$ are its waiting times.