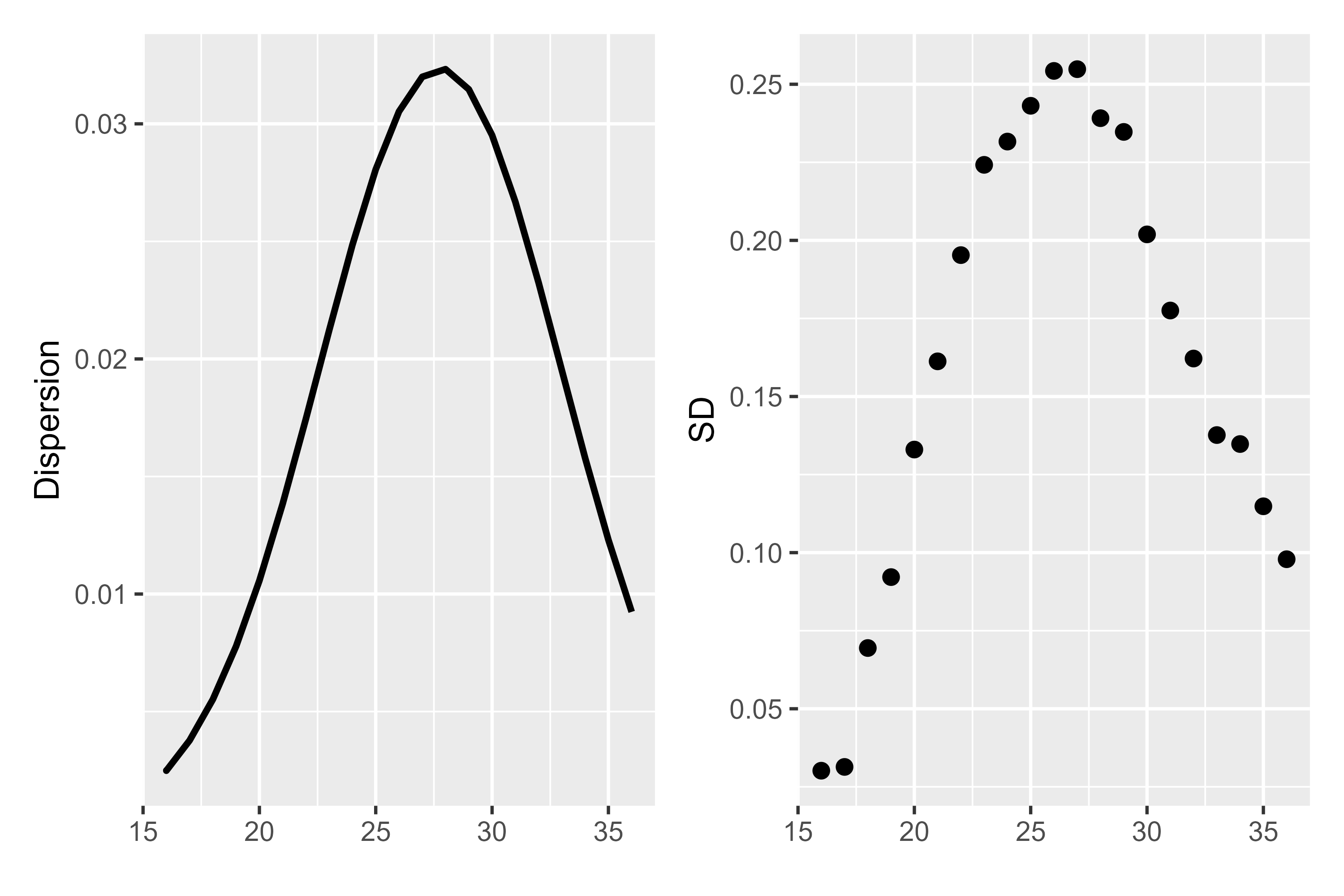
I'm unsure about the relationship between dispersion estimates (precision^-1) from beta regression models (log link) and the standard deviation.
The left panel is from a glmmTMB model ...
mod <- glmmTMB(
y ~ poly(x, 2) + (1 + poly(x, 2) || id),
data = df,
dispformula = ~ poly(x, 2),
family = beta_family(link = "logit"),
)
predict(mod, type = "disp")^-1
The right panel are standard deviations for different values of x
df %>%
group_by(x) %>%
summarise(sd = sd(x), .groups = "drop")
There is a correspondence between the two, as you would expect, since they both measure spread in the data. But how would you convert one to the other? Exponentiating the dispersion measure produces values in the range of 1.002488 and 1.032853.