When plotting two kernel desitiy estimates into the same frame
plot(density(all4[,3],kernel="epanechnikov"),col='red',ylim=c(0,0.4),xlim=c(-50,50))
lines(density(all2[,3],kernel="epanechnikov"),col="blue",ylim=c(0,0.4),xlim=c(-50,50))
the result looks like this:
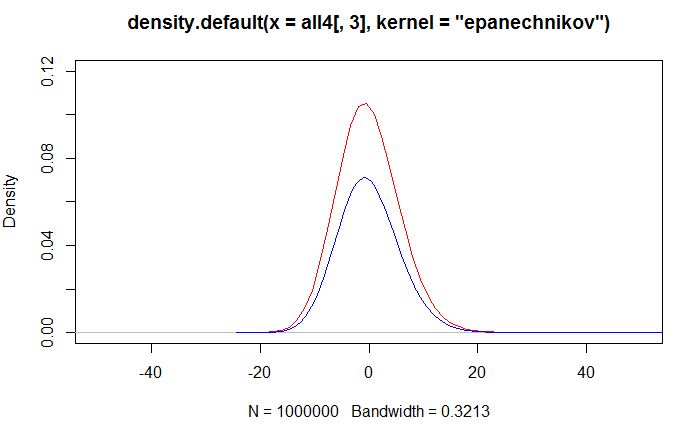
which is quite strange since the area under both plots should be unity. The data related to the red curve has an outlier (its maximum is 702.6).
> summary(all4[,3])
Min. 1st Qu. Median Mean 3rd Qu. Max.
-22.3100 -4.0940 -0.4151 -0.1533 3.4880 702.6000
> summary(all2[,3])
Min. 1st Qu. Median Mean 3rd Qu. Max.
-23.520 -4.041 -0.367 -0.109 3.533 67.780
After removing the outlier the curves match pretty well
a4 = sort(all4[,3])
a2 = sort(all2[,3])
a4 = a4[6:999995]
a2 = a2[6:999995]
plot(density(a4,kernel="epanechnikov"),col='red',ylim=c(0,0.2),xlim=c(-50,50),lwd=3)
lines(density(a2,kernel="epanechnikov"),col="blue",ylim=c(0,0.2),xlim=c(-50,50))
> summary(a4)
Min. 1st Qu. Median Mean 3rd Qu. Max.
-21.0200 -4.0940 -0.4151 -0.1541 3.4880 39.6700
> summary(a2)
Min. 1st Qu. Median Mean 3rd Qu. Max.
-21.9200 -4.0410 -0.3670 -0.1091 3.5330 32.2900
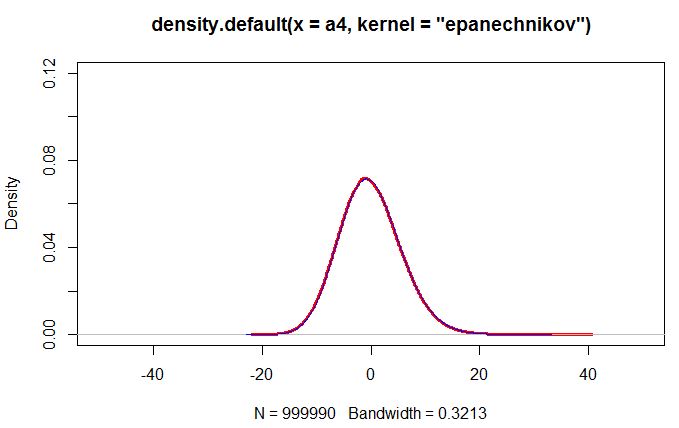
I am confused since the outlier should STEAL probability mass from the body of the distribution. But the red curve in the first picture seems to be inflated. Moreover, since both density curves are on the same scales, the area under both curves should be the same, also in the first plot. Where is my mistake?
EDIT: Following Momos recommendations I integrated over the densities. Indeed, the result for the weird one is far beyond unity!
library(sfsmisc)
dd1 = density(all4[,3],kernel="epanechnikov")
dd2 = density(all2[,3],kernel="epanechnikov")
integrate.xy(dd1$x,dd1$y)
[1] 1.490016
integrate.xy(dd2$x,dd2$y)
[1] 0.9977962

integrate. $\endgroup$integrateseems not to work sincedensity(some data)is not a function. I usedintegrate.xyinstead and there seem to be problems indeed! $\endgroup$