2
$\begingroup$

I have made several experiments, the result graphs are below, I need to figure out what is the distribution function of the following random variables... Where do I start with statistical analysis of my experiment?

How to perform these analysis with spss?

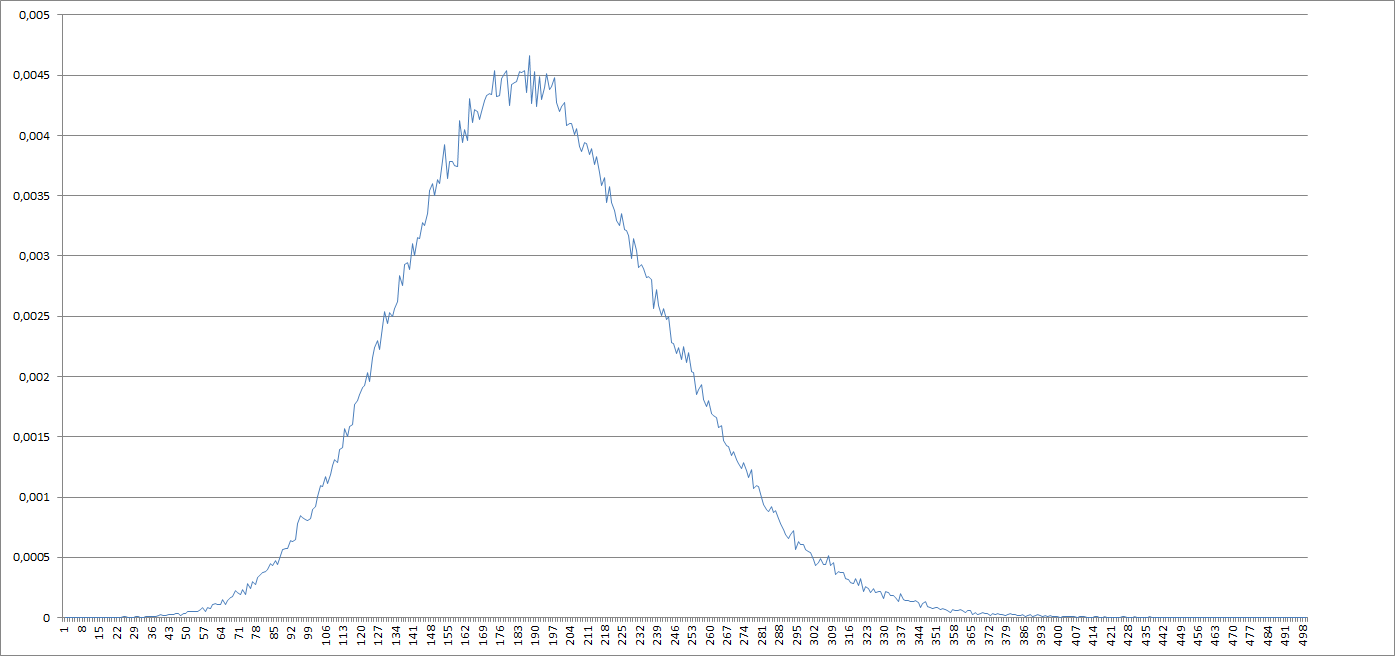
Sequence 1

Sequence 2

EDIT Explanations: I'm testing algorithm efficiency for 2 algorithms, the graphs describe efficiency distribution.

  1. I made 500000 tests for 2 algorithms, which produced 2 sets of 500000 floating point values.

  2. I've computed MIN and MAX value of the set (The smaller the values the better the result is, the bigger the values the worser the result is)

  3. I wanted to see the distribution of values according to 500 groups, so I've computed step = (MAX-MIN) / 500

  4. I've computed the amount of values that fall into each of 500 segments

[MIN + 0 * Step..MIN + 1 * Step],

[MIN + 1 * Step, MIN + 2 * Step], ...

[MIN + 498 * Step..MIN + 499 * Step]

The graph you see below represents on x-axis an index of the segment, on y-axis the amount values fallen into the segment divided by the total number of values, and also divided by the Step = (Max - Min) / 500. As a result this should represent the distribution function of my algorithm efficiency...

EDIT Here is DOC containing SPSS > Analyze > Descriptive Statistics > Explore output. Can anybody help reading that?

Improve this question
$\endgroup$
4
  • 3
    $\begingroup$ It might help if you explain the experiment. $\endgroup$
    – mark999
    Commented Jul 6, 2011 at 7:37
  • $\begingroup$ I've added the explenations $\endgroup$
    – Lu4
    Commented Jul 6, 2011 at 8:15
  • $\begingroup$ You have, in a roundabout way, created histograms of 500000 observations with 500 evenly spaced bins, and you want to know if there is a functional form you can use to summarize the results? $\endgroup$
    – fgregg
    Commented Jul 6, 2011 at 17:58
  • $\begingroup$ Yes, I need distribution function in analytical form. By having the function in analytical form it will be easier to compute average and other statistical properties of my experiment. I will not need to make 1000000 tests while having my computer running for a weak. Once having a law that describes the statistics, I will fit it into the data, estimate it's parameters and use those to compare results. $\endgroup$
    – Lu4
    Commented Jul 7, 2011 at 0:11

2 Answers 2

Reset to default
2
$\begingroup$

Your data describe how long it took before some event takes place, but with some overhead such that the event will never take place before some time $t$. Look into a shifted negative binomial distribution.

Improve this answer
$\endgroup$
0
$\begingroup$

In case somebody is interested, Skew normal distribution, it fits best

Read more at http://en.wikipedia.org/wiki/Skew_normal_distribution

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.