12
$\begingroup$

For a Normal distribution 'bell-shaped' curve, one would have thought that the height should have an ideal value. Knowing this value may be one quick indicator to check if the data is normally distributed.

However, I could not find its formal value. Most places, the shape is shown but not the y-axis measurements. http://www.stat.yale.edu/Courses/1997-98/101/normal.htm

In some graphs where it is mentioned, it is 0.4. http://en.wikipedia.org/wiki/File:Normal_Distribution_PDF.svg . But on the main page (http://en.wikipedia.org/wiki/Normal_distribution), value of 0.4 is not mentioned anywhere.

Is this the correct value and what is its mathematical basis? Thanks for your insight.

Edit:

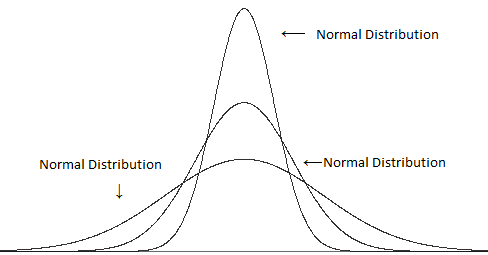
The three curves shown in @Glen_b 's answer and on the wiki page (with mean=0) have same mean but different SDs. All tests would show that no significant difference between them. But they are clearly from different populations. Which test can we then apply to determine the difference in standard deviations of two distributions?

I checked on net and found it to be the F-test.

But is there a specific name for a distribution curve that is similar to one with mean of 0 and standard deviation of 1 (and peak at 0.4)?

Answered by Aleksandr Blekh in comments: "standard normal distribution or the unit normal distribution denoted by N(0,1)".

However, it is not emphasized that, if the means are not different, F-test or the KS test (as suggested by Glen_b in the comments) should be done to determine if the standard deviations are different, indicating different populations.

Improve this question
$\endgroup$
7
  • $\begingroup$ It's not clear what function "bell shaped" serves in your question. A normal density has a bell shape (but one can have a distinctly-bell shaped density that's non normal). If you removed it, so the question just said "normal distribution", would that change the intent of the question? $\endgroup$
    – Glen_b
    Commented Mar 27, 2015 at 2:22
  • $\begingroup$ I meant height of the density curve of normally distributed data. $\endgroup$
    – rnso
    Commented Mar 27, 2015 at 2:31
  • 1
    $\begingroup$ Your claim "all tests would show no significant difference between them" is false. At reasonable sample sizes, an F test for variance (testing if the ratio of variances differs from 1) would find the difference easily, as would a simple Kolmogorov Smirnov test. $\endgroup$
    – Glen_b
    Commented Mar 27, 2015 at 3:16
  • $\begingroup$ I was thinking of all tests of comparing means, as is generally done. Thanks for your explanations. $\endgroup$
    – rnso
    Commented Mar 27, 2015 at 3:19
  • 1
    $\begingroup$ Re: your last question. Definition from corresponding Wikipedia article: "If $\mu = 0$ and $\sigma = 1$, the distribution is called the standard normal distribution or the unit normal distribution denoted by $N(0,1)$" (emphasis mine; the standard normal distribution is the one that peaks at ~0.4). $\endgroup$
    – Aleksandr Blekh
    Commented Mar 27, 2015 at 3:21

1 Answer 1

Reset to default
25
$\begingroup$

The height of the mode in a normal density is $\frac{1}{\sqrt{2\pi}\sigma}\approx \frac{.3989}{\sigma}$ (or roughly 0.4/$\sigma$). You can see this by substituting the mode (which is also the mean, $\mu$) for $x$ in the formula for a normal density.

So there's no single "ideal height" -- it depends on the standard deviation

edit: see here:
3 normal densities

Indeed the same thing can be seen from the wikipedia diagram you linked to -- it shows four different normal densities, and only one of them has a height near 0.4

A normal distribution with mean 0 and standard deviation 1 is called a 'standard normal distribution'

Improve this answer
$\endgroup$
5
  • $\begingroup$ So peakedness does not indicate normality or otherwise? Apologies of a very basic question. $\endgroup$
    – rnso
    Commented Mar 27, 2015 at 2:40
  • 2
    $\begingroup$ It depends on how you're defining 'peakedness'. If you mean "height of peak, without regard for relative spread" then no, as you can see from the diagram in your question, or the one in my answer. If you adjust for the spread (i.e. standardize), then all normal densities standardized to have $\sigma=1$ have the same height at the mode, but an infinite number of unimodal (but non-normal) distributions could have exactly the same height at the mode (it's trivial to construct one, for example via finite mixture distributions). $\endgroup$
    – Glen_b
    Commented Mar 27, 2015 at 2:47
  • $\begingroup$ Please see the edit in my question above. $\endgroup$
    – rnso
    Commented Mar 27, 2015 at 2:55
  • $\begingroup$ @Glen_b Where did you get the mode height formula from? I'm having trouble finding a derivation. $\endgroup$
    – tel
    Commented Nov 27, 2018 at 14:40
  • $\begingroup$ Never mind, I figured it out. You just set $x = \mu$ and find the value of the PDF. If you really want to, you can also confirm that $x=\mu$ is a maximum via differentiation, but in this case that seems overkill. $\endgroup$
    – tel
    Commented Nov 27, 2018 at 15:10

Not the answer you're looking for? Browse other questions tagged or ask your own question.