Well, I observe that the standard statistical software package tests regression coefficients if they are statiscally different from zero, that is, not specifically higher nor lower than zero -- a bilateral test in a normal distribution, for instance. But why is that? I understand that if your test rejects the null in a bilateral test, you'll rejected it even more significantly in a one-sided test. But you may risk not rejecting the null, when it should be rejected, in a bilateral test -- right? Is there any discussion on this topic on why not doing one-sided tests on the coefficients? Or not frequently, at least.
$\begingroup$
$\endgroup$
4
-
$\begingroup$ Your question is a little confusing in the last sentence. There may be a missing word, or perhaps more than one, and the form of the question (assuming I guess its meaning correctly at all) seems to contradict the immediately preceding point. Can you edit to clarify what you're asking there? $\endgroup$– Glen_bCommented Apr 1, 2015 at 1:40
-
$\begingroup$ Better? I think I managed to correct it. $\endgroup$– John DoeCommented Apr 1, 2015 at 1:52
-
$\begingroup$ How are you deciding which one-sided test to use? By looking at the data? $\endgroup$– Glen_bCommented Apr 1, 2015 at 5:25
-
$\begingroup$ Yes. I if it has a positive slope, one would use the right side of the distribution (as I understand). $\endgroup$– John DoeCommented Apr 1, 2015 at 12:48
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
The problem with choosing which direction you want to test for after you see the data is that your claimed significance level is a lie.
The probability that you'll reject the null when it's in fact true is doubled from the claimed (nominal) level. This is a form of data-dredging.
If the null hypothesis is true, half the time you'll have an observed slope greater than the slope in the null hypothesis and half the time it will be less... and of those cases that are far enough from the null-hypothesis slope to be significant, half are greater than it and half are less than it.
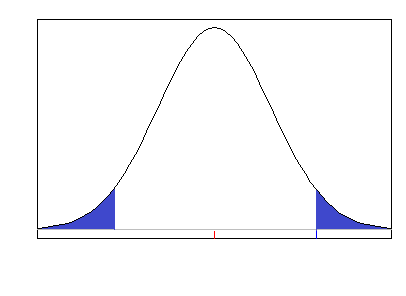
For a test at level $\alpha$, when the null is true, you have a probability of $\alpha/2$ in each tail.
If you choose which side to test on by looking at the data, it's instead like this:
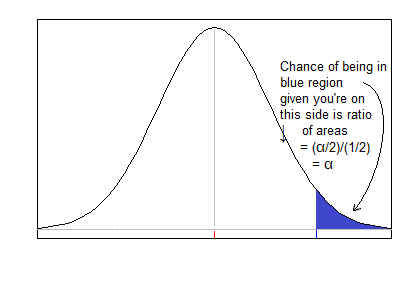
-- as I suggested, it doubles the significance level.
For a one sided test to have the claimed properties, you have to specify the event of interest before you see it.
If you want to be able to specify it after the fact, to do it with the correct significance level, you in effect end up doing a two-tailed test.
