I have been working on a question for hours now and I thought I would ask stack exchange.
"Deer ticks can be carriers of either Lyme disease or human granulocytic ehrlichiosis (HGE). Based on a recent study, suppose that 16% of all ticks in a certain location carry Lyme disease, 10% carry HGE, and 10% of the ticks that carry at least one of these diseases in fact carry both of them. If a randomly selected tick is found to have carried HGE, what is the probability that the selected tick is also a carrier of Lyme disease?"
The answer is .236, and I was wondering if someone could tell me how that is. I'm convinced that the answer is .1 - "10% of the ticks that carry at least one of these diseases in fact carry both of them." So, what more information is needed than that sentence? The tick has HGE, and .1 of ticks that carry a disease carry another. So why isn't the answer simply .1? Thanks for your help in advance.

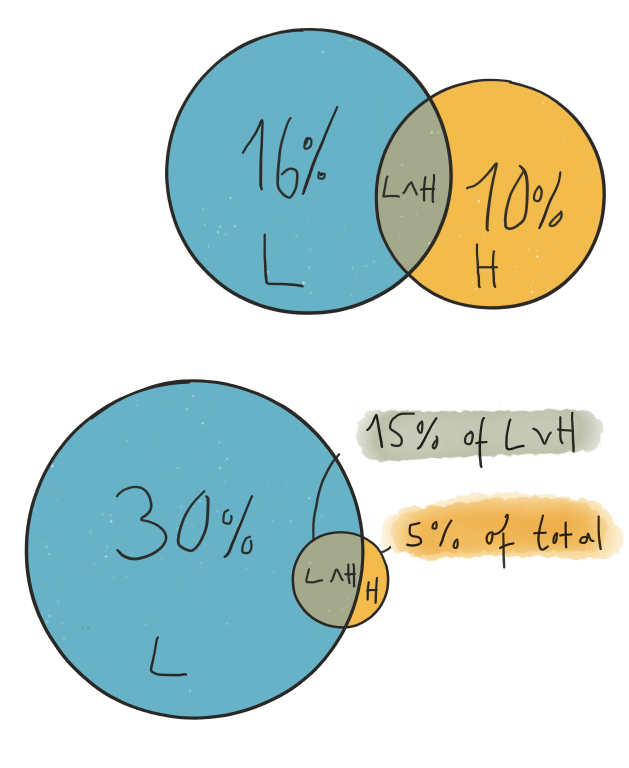
self-studytag to your question. See stats.stackexchange.com/tags/self-study/info $\endgroup$