2
$\begingroup$

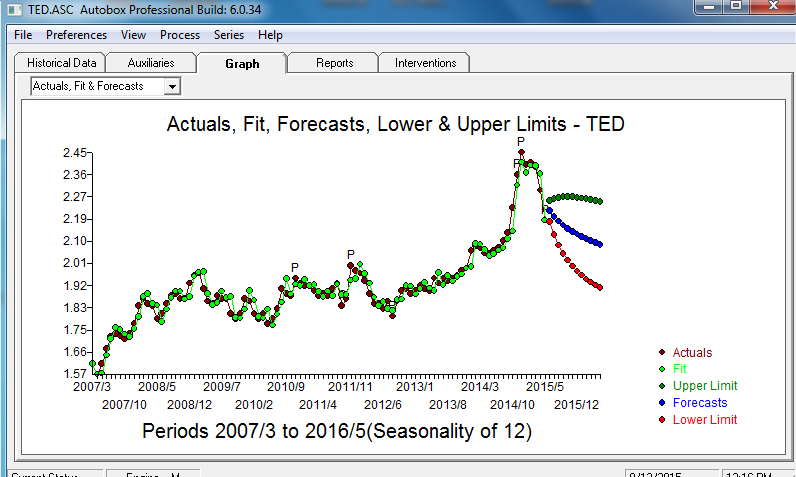
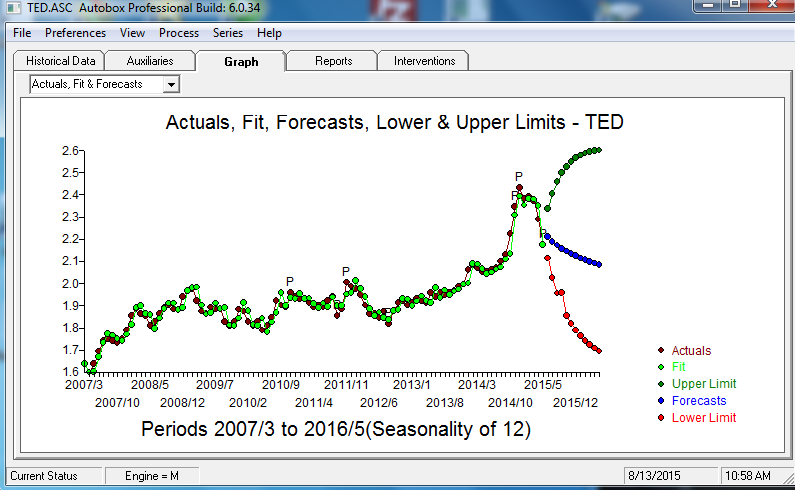
I am very new to time-series analysis and have got some time-series data regarding product prices. The data set is monthly data collect since 1993 to 2014.

I have tried plotting the ACF and PACF but I do not really understand the meaning behind these plots. Furthermore, I am not sure if I need to convert the data series by differencing of order 1, then proceed to plot ACF and PACF.

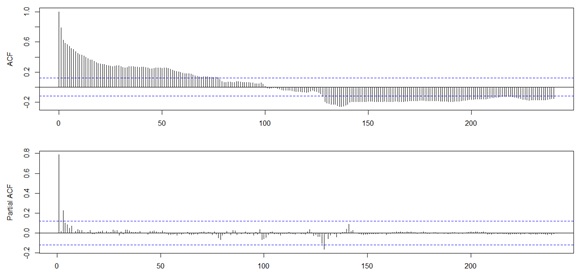
My ACF and PACF plot

I plot a lag.max of 250, since there are alot of data point, and from the ACF, there appears too many lag that are above the confidence interval. However, for PACF, there are only 2 lag that are above the condidence interval.

What is the meaning behind this? Or do I need to do differencing before the acf plot? In addition, how do I further evaluate my data in time-series plot?

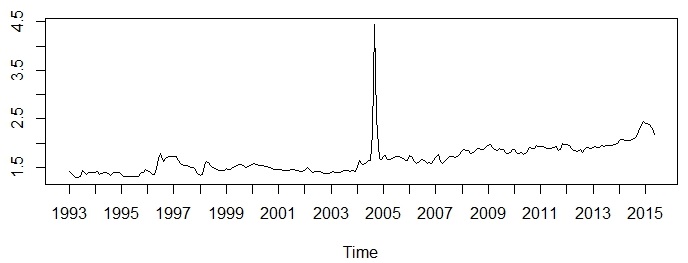
My Data Set plot

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

The ACF and PACF are descriptive statistics showing simple correlation and conditional correlation. They are sometimes useful in identifying ARIMA models that 1) have no Pulses (your series does) and 2) have no deterministic time trends or level shifts ( your series seems to have no trend followed by a period that has trend) and whose parameters and model error variances are constant over time. If you actually post your data in an excel format I will try and help you understand more BUT my initial visual assessment is that this is a time series that may have complications/opportunities that will challenge simple (1960 type !) ARIMA analysis or simple model selection (list-based) schemes that use an AIC/BIC criteria to select/identify a model. We should aspire to keeping things/models simple but not too simple so much so that they are of little value !

EDIT AFTER RECEIPT OF DATA:

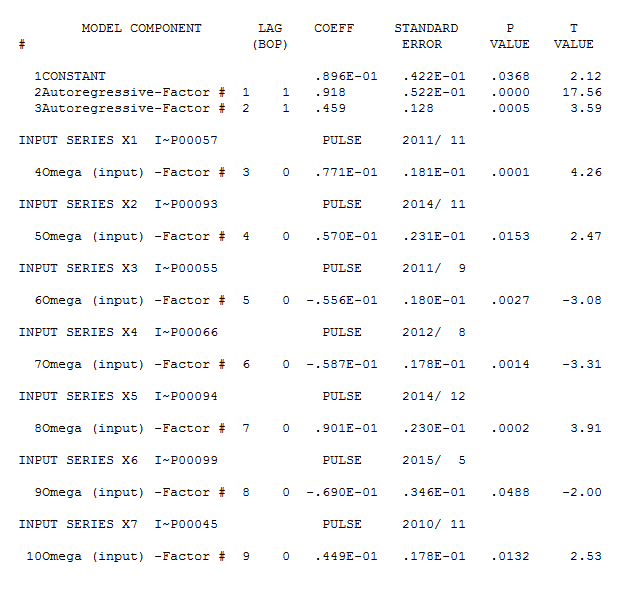
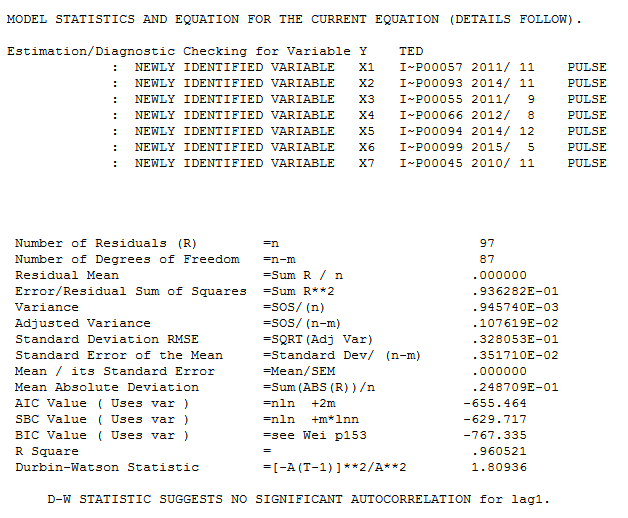
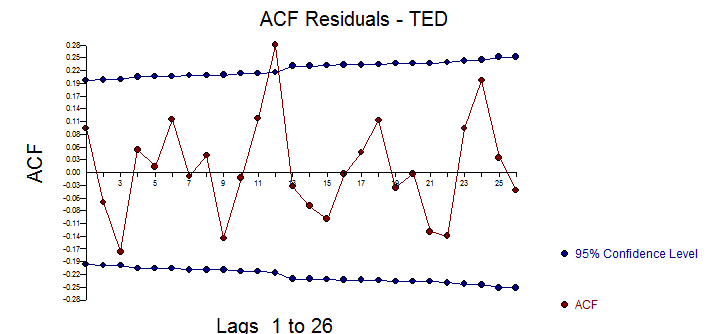
I took the 269 monthly values and analyzed it with the automatic option in AUTOBOX. After identifying a global model taking into account various pulse effects a significant difference was found between the first 169 values and the most recent 99 values enter image description here . This is visually obvious.The best model for the most recent 99 values was enter image description here and here enter image description here . The residuals from the model appear well behaved [enter image description here] . [4] The ACF of the residuals suggest a slight possibility of a minor seasonal effect but most likely not important .enter image description here . The plot of the actual , fit and forecast using standard ARIMA procedures tp compute uncertainty limits is here enter image description here . Note however from http://www.autobox.com/cms/index.php/blog/entry/you-should-have-50-confidence-in-your-confidence-limits that the forecast limits are flawed on two accounts. Taking into account the possibility of future values being effected by pulses i.e.unusual values and that the estimated parameters are not necessarily the poulation parameters we get a more realistic picture enter image description here

Improve this answer
$\endgroup$
13
  • $\begingroup$ filedropper.com/product_1 $\endgroup$
    – Ted
    Commented Aug 13, 2015 at 10:17
  • $\begingroup$ The above is the file link as I do not have enough rep points to post it on my question. I have a few questions to ask as well. So to see the pulses, we just look at the data set to see if it fluctuates across the yearly data series? And to see the model error variance, you check whether the differences/residual are "slightly constant/fluctuates" along 0? $\endgroup$
    – Ted
    Commented Aug 13, 2015 at 10:30
  • $\begingroup$ To detect pulses you build an ARIMA model and then follow TSAY unc.edu/~jbhill/tsay.pdf and the flow diagram in autobox.com/cms/index.php/blog/entry/…. To tests for constant error variance you evaluate alternative break-points as detailed by TSAY $\endgroup$
    – IrishStat
    Commented Aug 13, 2015 at 11:34
  • $\begingroup$ As I do not have Autobox, and the version that can be downloaded is a demo version, so through using R, how do I identify structural break in the series? By structural break, does it means that for the first 170 observations there are no trend, thus it is side aside? Through the use of ACF and PACF, can I detect pulses as well? Do I need to do differencing in my data, as it seems that there are alot of points that falls outside the Confidence interval in the ACF? or Since there are only 2 points that falls outside the Confidence interval in PACF, It means there is no need for differencing. $\endgroup$
    – Ted
    Commented Aug 14, 2015 at 1:06
  • $\begingroup$ Structural breaks ( detecting a change in parameters over time)requires identify an appropriate ARIMA model and Intervention series.Use the intervention series to cleanse the data of pulses,level shifts,time trends.Take the adjusted series and estimate parameters for different time sections. Find the greatest difference in parameters in terms of two distinct sections and conclude that the parameters have changed over time. That would be 1-169 and 170-269 . Now take the observations from 170-269 and model them in terms of an ARIMA model and Intervention series. $\endgroup$
    – IrishStat
    Commented Aug 14, 2015 at 2:13

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.