0
$\begingroup$

What is the expected value of the expected of x conditional of y? $E(E(X|Y))$ and $E(E(X^2|Y))$?

I was doing this question. And got this result. But got stumped at the next question. Which asks me to find the expected value of the conditional probability.

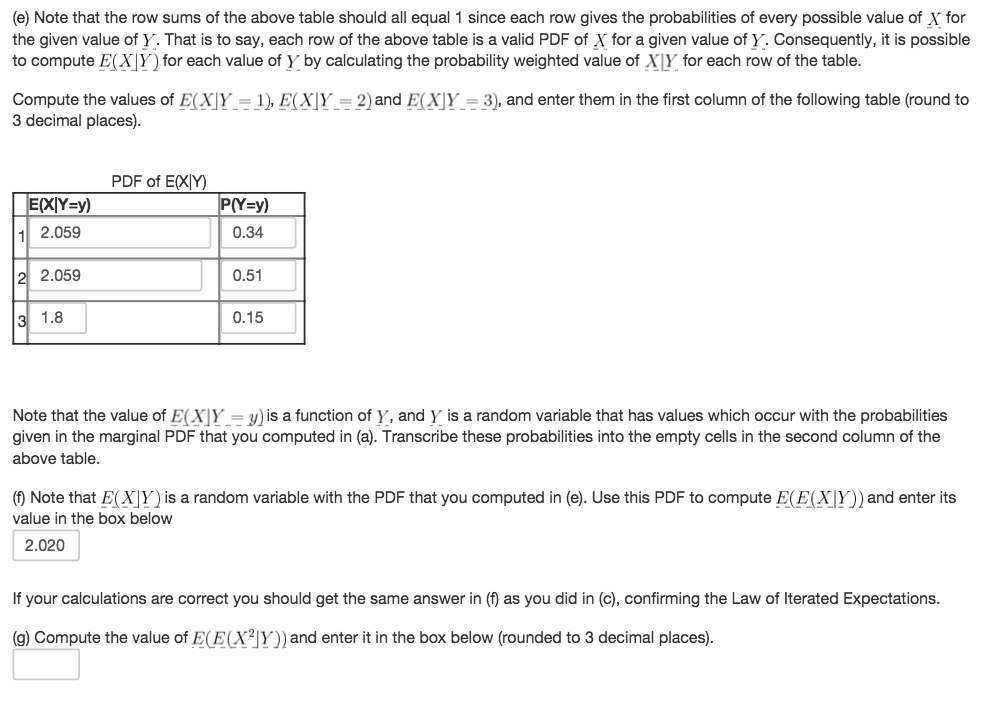
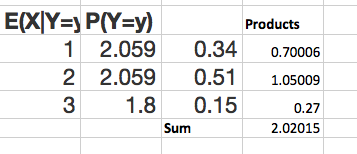
enter image description here

I guessed and computed it like this. Getting $E(E(X|Y)) = 2.020$

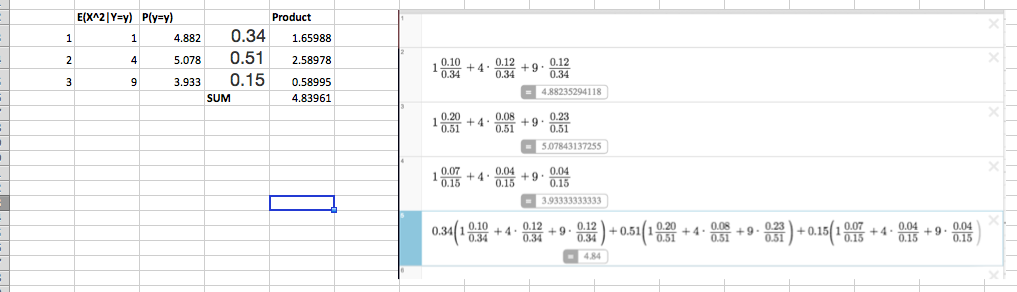
enter image description here

I guessed and got $E(E(X^2|Y))= 4.840$

enter image description here

Improve this question
$\endgroup$
4
  • 5
    $\begingroup$ Maybe you could clean up a bit and remove these non-self explanatory Excel screen shots and replace them with two lines of computations each. $\endgroup$
    – sheß
    Commented Sep 4, 2015 at 8:59
  • $\begingroup$ But sheb they explain my working out. I don't think it's better to include some form of evidence of effort and reasoning. $\endgroup$
    – Ivan
    Commented Sep 5, 2015 at 13:22
  • $\begingroup$ It's far easier to read a calculation in $\LaTeX$ than it is to read an Excel sheet. $\endgroup$
    – Silverfish
    Commented Nov 7, 2015 at 13:41
  • 1
    $\begingroup$ The characterization in this question is not quite right: "$E[E[X^2|Y]]$" asks not for the expectation of a "conditional probability"; rather, it asks for the expectation of a random variable (namely, the variable $E[X^2|Y]$). There's nothing new in that: the calculation is carried out exactly as it would be with any random variable. $\endgroup$
    – whuber ♦
    Commented Nov 7, 2015 at 14:55

1 Answer 1

Reset to default
4
$\begingroup$

Your parentheses do not match, almost nowhere, this makes your question ambiguous. If you mean $E_Y(E_X(X|Y))$, then your answer is the law of iterated expectations.

Basically $E_Y(E_X(g(X)|Y))=E(g(X))$

Improve this answer
$\endgroup$
2
  • $\begingroup$ So it's just E[E(X^2|Y)] = E(X^2) = 1*0.34 + 4*0.51+9*0.15? which is also = 4.841 And E[E(X|Y)] = E(X) = 1*0.34 + 2*0.51+3*0.15 which is also 2.020 $\endgroup$
    – Ivan
    Commented Sep 4, 2015 at 8:44
  • $\begingroup$ Sheb? responds plox how's this? $\endgroup$
    – Ivan
    Commented Sep 5, 2015 at 13:20

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.