0
$\begingroup$

This may be an elementary question. Say we have a univariate continuous random variable $X$ with unknown pdf $p(x)$, $ \forall x \in X$.

Presumably we can assign a second probability measure $Q(p)$ to the set of all possible realizations of the pdf $p$, define a Radon-Nikodym derivative etc.

Do these types of "hyper-distributions" have a general name? I do realize you can define distribution parameters probabilistically, I am looking for something more non-parametric.

P.S. Please let me know if my notation is incorrect!

Edit: There are examples of such distributions (see e.g. https://en.wikipedia.org/wiki/Dirichlet_process), but I am looking for a more general theory.

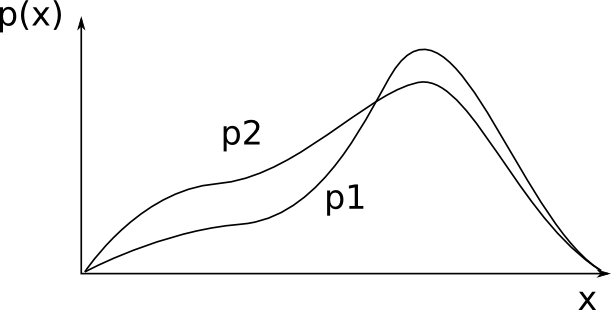
In case it helps, below is an example:

Two possible pdf

This is a simplified discrete case. Say we have two pdf's $p_1$ and $p_2$, where each has probability 0.5 under $Q$. While you can easily marginalize out the index (i.e. the $i \in \{1, 2\}$), I am interested in the distribution of possible pdf's.

Improve this question
$\endgroup$
8
  • $\begingroup$ I think you are thinking of level set of probability distributions. This paper may be helpful: pelletierb.perso.math.cnrs.fr/Publications_files/cpp-jnps.pdf $\endgroup$
    – John Jiang
    Commented Sep 21, 2015 at 4:57
  • 1
    $\begingroup$ Are you perhaps referring to a (Bayes) prior distribution? Your description is not clear, because "assign a second probability measure to the set of all possible values of the pdf $p$" could be interpreted liberally, in the sense of a probability distribution over the set of all univariate distribution functions, or literally, in the sense that identifies "all possible values" with $\mathbb R$. Which is it? $\endgroup$
    – whuber ♦
    Commented Sep 21, 2015 at 14:18
  • $\begingroup$ @whuber Apologies about the description, I have added some more detail. I am interested in the former of the two options you describe ("probability distribution over the set of all univariate distribution functions"). They are indeed some kind of Bayesian prior distribution, but I am looking for a more specific term I can search for :) $\endgroup$
    – user157969
    Commented Sep 22, 2015 at 3:13
  • 1
    $\begingroup$ I'm not sure if this applies, but it sounds a lot like what goes on in a Bayesian hierarchical model where we have some data distributed as some distribution, but at least some of those parameters are unknown, so we place a prior over the parameters themselves and the model has several layers. $\endgroup$
    – Sycorax ♦
    Commented Sep 22, 2015 at 3:38
  • $\begingroup$ @user777 Thanks, yes I think you on the right track - in fact what I am thinking of could be described as a "nonparametric hyperprior". $\endgroup$
    – user157969
    Commented Sep 22, 2015 at 5:02

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.