Histogram or box plot, to compare two distributions of means?

Question

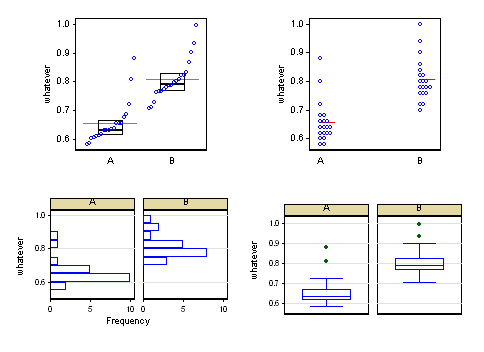
I've got two different distributions of participant reaction time means (20 data points for condition A, another 20 for condition B). I'm stuck trying to decide between using a histogram, or a box plot to display the differences in the distribution. I'd appreciate any opinions on the matter.

EDIT
Here's the data, in case you want to play around with it / offer suggestions:

A = [0.87923964999999993, 0.58451829999999994, 0.80928291666666674, 0.68699328333333343, 0.72234159999999992, 0.65511489999999983, 0.65493080000000015, 0.65603638333333325, 0.60245800000000005, 0.6110293166666666, 0.63731213333333325, 0.62952980000000003, 0.61440959999999989, 0.67764006666666687, 0.61654928333333325, 0.60551748333333311, 0.63183041666666673, 0.63157981666666674, 0.63295355000000009, 0.58176853333333312]

B = [0.99643063333333315, 0.72695231666666671, 0.93434573333333326, 0.90142753333333336, 0.78560038333333337, 0.82246491666666666, 0.86708678333333333, 0.77973181666666647, 0.82362093333333319, 0.83155454999999989, 0.77494198333333331, 0.80204511666666667, 0.76222439999999991, 0.80875108333333323, 0.79778146666666672, 0.76476253333333311, 0.76522888333333339, 0.70649123333333341, 0.71163546666666666, 0.78614491666666664]

Answer 1

Thanks for posting the data. Here are four of several possibilities.

Top left is a quantile-box plot, a hybrid of quantile plot (points shown in order) and box plot. In addition to boxes showing medians and quartiles, extra horizontal lines show means. Both distributions show slight positive (upward, but conventionally called right) skewness.

Top right is a dot plot or strip plot with stacking (a little arbitrarily with bin width 0.02) and means superimposed.

Bottom left are histograms with arbitrary bin width 0.05 and bin limits multiples of the same.

Bottom right are conventional box plots with the Tukey rule of showing points separately that are more than 1.5 IQR from the nearer quartile. That rule selects two points in each case. Those two points are not the only points that straggle a little, but it takes the first two plots to make that really clear.

These designs are not exhaustive, even less definitive, and elements could be added to or subtracted from each.

As in quantum physics and certain kinds of philosophy, superficially contradictory statements are both correct:

1. The example data are rather well behaved with at most one awkward feature to note, the slight skewness. In these circumstances, all graphs show the main message faithfully and without serious distortion.

2. There is still room for argument among close competitors about which design is best. My personal bias is to designs showing all the data so that I can decide for myself what is trivial noise, what is interesting fine structure and what are clear signals. When it's somebody else's data, I often distrust the graph designs chosen, as when for example there is interest in means and people show box plots (but not means!).

The next possibility I would try given infinite energy would be a quantile-quantile plot for both groups with a normal (Gaussian) distribution, as suggested by @EngrStudent.

In this case a transformation, e.g. to logarithms or reciprocals, might be contemplated, but as the data vary less than twofold, the effect might be modest.

Answer 2

The decision is yours entirely as there isn't a "hard and fast" rule to which you should adhere. However, you should be cognizant of a couple of things.

The histogram will provide more information as to the shape of the underlying data as you will be able to see the height of individual bars. From that perspective, a histogram may be better.

On the other hand, the shape of a histogram is largely affected by which bins (the categories along the $x$-axis) you select. For example, if your data spans 0 to 20 on the $x$-axis, you could choose to have two bars with bins spanning from 0 to 10 and 10 to 20 or you could choose four bars with bins from 0 to 5, 5 to 10, 10 to 15, and 15 to 20. There are an infinite number of choices you could make there and there is no rule for how many bins you should have. There are no "bins" to select when working with a boxplot and thus there is no way for you to skew the appearance of your results to look favorable to what you are trying to do.

I believe the decision comes down to flexibility and know-how. If you want something that is flexible and relies on your subject-specific knowledge, I think a histogram is the way to go. If you want something that doesn't require any tuning or that you feel you don't know enough about to make a good decision on widths of bins, etc. then I would opt for a boxplot.