Comparing two models through ANOVA with different types of functions

Question

See this file here: Decay.TXT.

I first tried to fit the logarithmic model first

dec = read.table('decay.txt', header=T)
attach(dec)
logy <- log(y)
model1 <- lm(logy ~ x)

And now I try to fit a quadratic model into the data:

model2 <- lm(y ~ x + I(x^2))

I now attempt to get the r^2 (r-squared values) of the models.

(rsq1 <- summary(model1)$r.squared)
[1] 0.8307964

(rsq2 <- summary(model2)$r.squared)
[1] 0.9079788

Obviously as seen, here the coefficient of determination of the quadratic regression model is better than the exponential regression model (for this dataset).

However, the critical appraisal of these models says otherwise. For the exponential model, we see that:

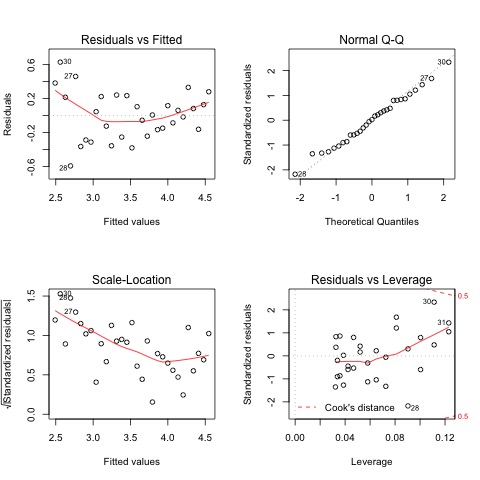
And for the quadratic polynomial model, we see that:

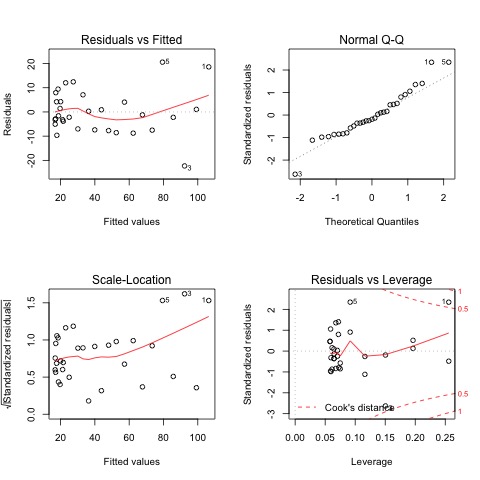
The QQ Plot reveals something strange with the residuals for the Quadratic regression i.e "Not Normally Distributed".

Now I am trying to use ANOVA, to compare the two models. And I type:

anova(model1, model2)  

and there is an error regarding the missing variable y. What is the right way to conduct the anova ?

Answer 1

The two models cannot be compared using anova() because the response variable is different (y vs. logy).

There are similar questions in the cloud: How to use anova for two models comparison?

You can compare:

model1 <- lm(logy ~ x)
model2 <- lm(logy ~ x + I(x^2))

anova(model1, model2) should tell if the model with the second order polynomial differ from the simple linear model.