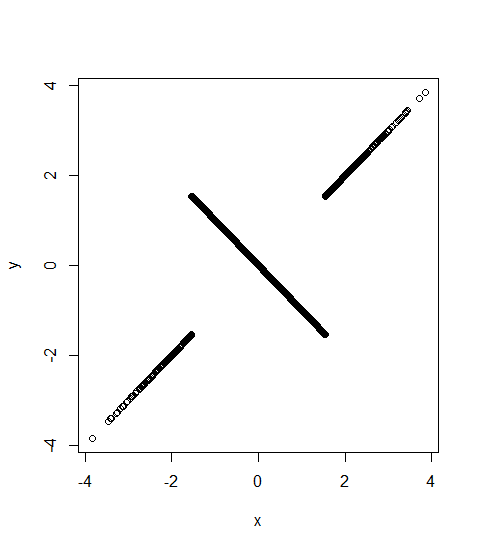Any example of (roughly) independent variables that are dependent at extreme values?

Question

I am looking for an example of 2 random variables $X$, $Y$ such that

$$\newcommand{\cor}{{\rm cor}}|\cor(X,Y)| \approx 0 $$

but when consider the tail part of the distributions, they are highly correlated. (I try to avoid 'correlated' / 'correlation' for the tail because it might not be linear).

Probably use this:

$$|\cor(X', Y')| \gg 0$$

where $X'$ is conditional on $X > 90\%$ of $X$'s population, and $Y'$ is defined in the same sense.

Answer 1

Here's an example where $X$ and $Y$ even have normal marginals.

Let:

$$X \sim N(0,1)$$

Conditional on $X$, let $Y = X$ if $|X| > \phi$, or $Y = -X$ otherwise, for some constant $\phi$.

You can show that, independently of $\phi$, marginally we have:

$$Y \sim N(0,1)$$

There is a value of $\phi$ such that $\text{cor}(X,Y) = 0$. If $\phi = 1.54$ then $\text{cor}(X,Y)\approx 0$.

However, $X$ and $Y$ are not independent, and extreme values of both are perfectly dependent. See simulation in R below, and the plot that follows.

Nsim <- 10000
set.seed(123)

x <- rnorm(Nsim)
y <- ifelse(abs(x)>1.54,x,-x)

print(cor(x,y)) # 0.00284 \approx 0

plot(x,y)

extreme.x <- which(abs(x)>qnorm(0.95))
extreme.y <- which(abs(y)>qnorm(0.95))
extreme.both <- intersect(extreme.x,extreme.y)

print(cor(x[extreme.both],y[extreme.both])) # Exactly 1