1
$\begingroup$

I am estimating a structural VAR Model in levels with $p=3$ and plot orthogonalised impulse response functions.

A structural VAR with p lags (sometimes abbreviated SVAR) is

$B_0 y_t = c_0 + B_1 y_{t-1} + B_2 y_{t-2} + \cdots + B_p y_{t-p} + \epsilon_t,$

where c0 is a k × 1 vector of constants, Bi is a k × k matrix (for every i = 0, ..., p) and $\epsilon_t$ is a k × 1 vector of error terms. The main diagonal terms of the B0 matrix (the coefficients on the ith variable in the ith equation) are scaled to 1.

The error terms $\epsilon_t$ (structural shocks) satisfy 3 conditions in the definition above, with the particularity that all the elements off the main diagonal of the covariance matrix $\mathrm{E}(\epsilon_t\epsilon_t') = \Sigma$ are zero. That is, the structural shocks are uncorrelated.

Afterwards, I am testing the data for co-integration and find $r=3$ co-integration relationships. This, will be used in modelling a VECM using $p=3$. The variables are $R\&D_1,R\&D_2, Capital, Labour, P_E, Output $ where $R\&D_x$ are research and development investments and $P_E$ is the electricity price. Now we want to analyse the impact of shocks to each variable in an impulse response function framework. The results look as following:

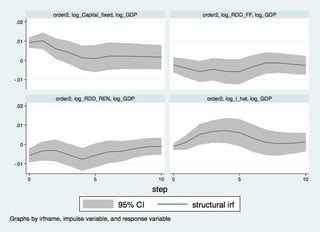
orthogonalised IRF based on VAR(3) in levels

orthogonalised IRF based on VECM(3)

While the shape of the 4 functions in general are the same I am very confused by the permanent nature of all the VECM IRFs. According to Lütkepohl's "New Introduction to multiple time series analysis" there should be at most 3 transitory effects as $r=3$, but to me it seems that there are no transitory effects in place. Further, I am wondering if this comparison enables me to conclude that my VAR in levels is correctly specified and co-integration does not cause any bias.

Thanks in advance!

Improve this question
$\endgroup$
4
  • $\begingroup$ Why do you have the same lag order in levels-VAR as in VECM? They should differ by 1 (at least for the reduced-form VAR, not so sure about SVAR, though). $\endgroup$
    – Richard Hardy
    Commented Nov 12, 2016 at 14:04
  • $\begingroup$ @RichardHardy The question was out of left field-I just added a missing context to it. You seem to understand this better, so edit it, please. $\endgroup$
    – Carl
    Commented Nov 12, 2016 at 16:01
  • $\begingroup$ @ RichardHardy, yes exactly the lag selection differs for the level VAR and the VECM as the VECM has its short run equations in first differences. Thus, lags in the VECM are 2, even though the insert command defines 3, and the baseline level var model is 3 lags. $\endgroup$
    – Raphael
    Commented Nov 15, 2016 at 13:57
  • $\begingroup$ Further, I was wondering, if the variables are given in logs, can impulse response functions then be interpreted in percentage deviations along the response path? $\endgroup$
    – Raphael
    Commented Nov 15, 2016 at 13:58

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.