2
$\begingroup$

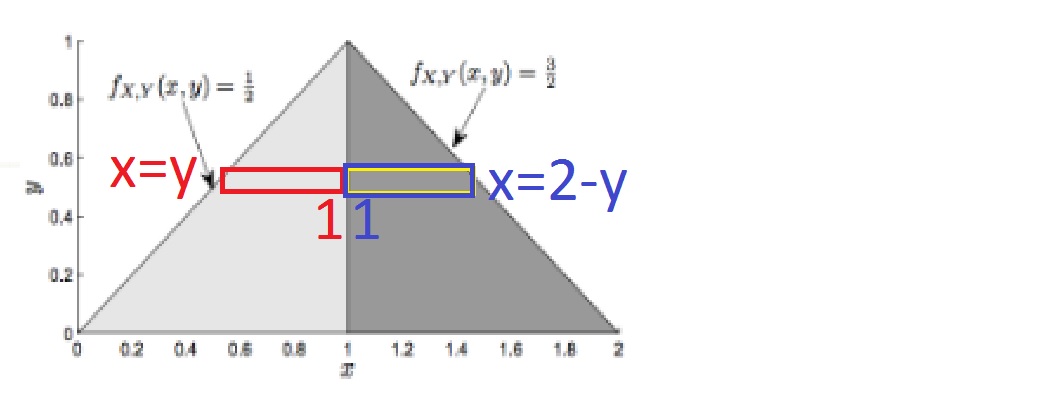
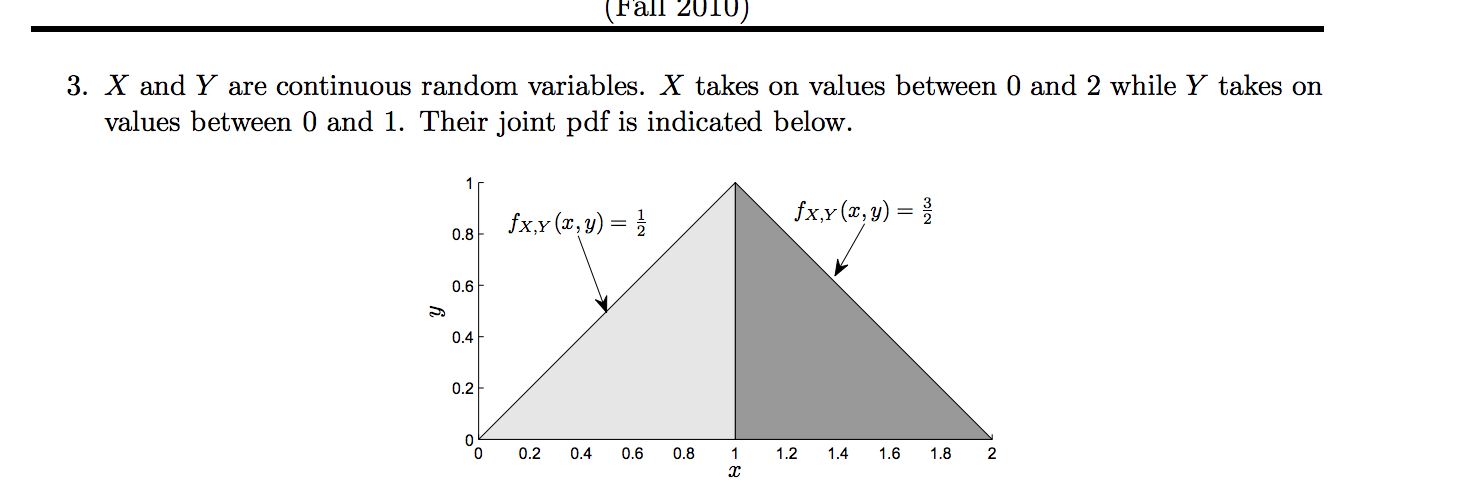
I am trying to learn probability theory through self study, so I have been working through the MIT open courseware problems. I am pretty stuck on the following one enter image description here

How do we find the marginal pdf of $f_Y(y)$? I know we need to integrate the joint over $X$ but I don't see how to do it?

Source: https://ocw.mit.edu/courses/electrical-engineering-and-computer-science/6-041-probabilistic-systems-analysis-and-applied-probability-fall-2010/assignments/MIT6_041F10_assn05.pdf

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ Since this is self study you need the self study tag. Then we can provide hints to help you. $\endgroup$
    – Michael R. Chernick
    Commented Feb 11, 2017 at 16:00

1 Answer 1

Reset to default
6
$\begingroup$

The following image is the ranges of integration:

$p_Y(y) = \int_x p_{X,Y}(x,y) \, \mathrm{d}x$ $= \int_1^{2-y} \ 3/2 \ dx+\int_y^{1} \ 1/2 \ dx $

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.