1
$\begingroup$

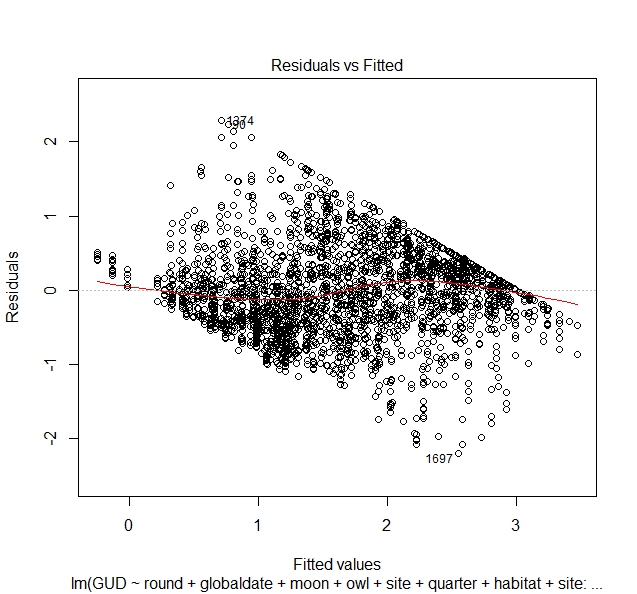
I have a dataset where the response variable is the amount of food left in a tray by the focal animal, in grams. Because of that, the response variable can only take values between 0 and 3 g, the amount of food offered.

When we look at the graph of residuals x fitted, the residuals are not behaving like a rectangle parallel to the x axis. If my understanding is correct, that implies my model is does not have homogeneity of variances. Am I interpreting this right?

enter image description here

From looking at the graph this potential heteroskedasticy looks like a result of the upper and lower limit. Fitted values close to 3 cannot have positive residuals because it would bring the response to above 3. The opposite happens on fitted values close to 0. No negative residuals are possible. Since this likely to happen in any bounded data, does it mean that all data with upper ad lower limits are heteroskedastic?

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ A more serious consequences of limited dependent variables is that OLS is generally no longer consistent, see, e.g., discussions of the Tobit model. $\endgroup$
    – Christoph Hanck
    Commented May 5, 2017 at 12:47
  • $\begingroup$ I looked on the Tobit model, it gives me impression that it is for censored data (when the actual value is 3.5 but I annotate >3). Would it still be relevant even though a value >3 cannot be observed? $\endgroup$
    – JMenezes
    Commented May 5, 2017 at 13:20
  • 2
    $\begingroup$ Yes, when the dependent variable is bounded in some interval, then a linear regression will ultimately (i.e., for certain x values) produce a fit outside that interval, which won't make sense. $\endgroup$
    – Christoph Hanck
    Commented May 5, 2017 at 15:01

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.