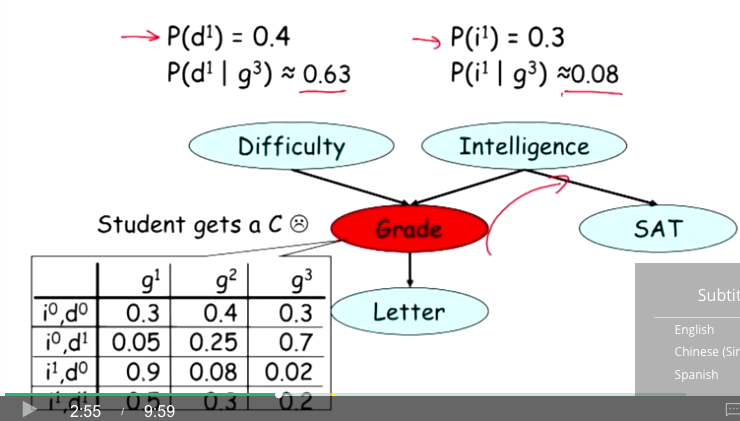
I have a feeling you may have misunderstood the question the slide was asking, (or rather, whether the slide was asking a question in the first place) and in fact the four probabilities stated there are "given" as an introduction to a problem (presumably continued in the next slide), rather than "the answers". Was there a question further down the line? (e.g. what is the probability the student gets a g3 grade irrespective of their intelligence and difficulty?). If not, I will admit that this question is causing me considerable confusion as well!
I'll explain my skepticism. If these are given
\begin{array}{rclclrcll}
P ( d^1) & = & 0.4 & ~~~\Rightarrow~~~ & P ( d^0) & = & 1 - 0.4 & = & 0.6 \\
P ( i^1) & = & 0.3 & ~~~\Rightarrow~~~ & P ( i^0) & = & 1 - 0.3 & = & 0.7
\end{array}
but $p(d^1 | g^3)$ and $p(i^1 | g^3)$ are not, and you're asked to prove that those are the 'correct' values, then in the absence of any further information we cannot establish that, unless there's an assumption of independence_ for $d$ and $i$, in which case:
\begin{array}{| c | c | c | c |}
\hline
& \mathbf{d^0} & \mathbf{d^1} & \mathbf{Column Totals} \\
\hline
\mathbf{i^0} & 0.42 & 0.28 & \mathbf{0.7} \\
\hline
\mathbf{i^1} & 0.18 & 0.12 & \mathbf{0.3} \\
\hline
\mathbf{Row Totals} & \mathbf{0.6} & \mathbf{0.4} & \mathbf{4}\\
\hline
\end{array}
Then here's the full table of joint probabilities:
\begin{array}{| c | c | c | c | c |}
\hline
& \mathbf{g^1} & \mathbf{g^2} & \mathbf{g^3} & \mathbf{Cols} \\
\hline
\mathbf{i^0}, \mathbf{d^0} & 0.3 \times 0.42 = 0.126 & 0.4 \times 0.42 = 0.168 & 0.3 \times 0.42 = 0.126 & \mathbf{0.42} \\
\hline
\mathbf{i^0}, \mathbf{d^1} & 0.05 \times 0.28 = 0.014 & 0.25 \times 0.28 = 0.07 & 0.7 \times 0.28 = 0.196 & \mathbf{0.28} \\
\hline
\mathbf{i^1}, \mathbf{d^0} & 0.9 \times 0.18 = 0.162 & 0.08 \times 0.18 = 0.0144 & 0.02 \times 0.18 = 0.0036 & \mathbf{0.18} \\
\hline
\mathbf{i^1}, \mathbf{d^1} & 0.5 \times 0.12 = 0.06 & 0.3 \times 0.12 = 0.036 & 0.2 \times 0.12 = 0.024& \mathbf{0.12} \\
\hline
\mathbf{Rows} & \mathbf{0.362} & \mathbf{0.2884} & \mathbf{0.3496} & \mathbf{1} \\
\hline
\end{array}
Therefore
- $ p ( d^1 |~ g^3 ) = p ( d^1, i^0 |~ g^3 ) + p ( d^1, i^1 |~ g^3 ) = 0.196 + 0.024 = 0.22 $
- $ p ( i^1 |~ g^3 ) = p ( i^1, d^0 |~ g^3 ) + p ( i^1, d^1 |~ g^3 ) = 0.0036 + 0.024 = 0.0276 $
Which are not the values we 'expected'.
Which leads me to believe those are also "given information", and that $i$ and $d$ are not independent, and that you're instead supposed to use that information to derive the true probability table between $i$ and $d$ (which you might then use to answer the real question, such as the one I posed in the introduction to this answer).
If this is the case, then the true joint probability table for $i$ and $d$ is given by the following system of equations:
$p(d^0, i^0 | g^3) + p(d^0, i^1 | g^3) = 0.37~~~~$ (from 1 - 0.63)
$p(d^1, i^0 | g^3) + p(d^1, i^1 | g^3) = 0.63~~~~$ (given)
$p(d^0, i^0 | g^3) + p(d^1, i^0 | g^3) = 0.92~~~~$ (from 1 - 0.08)
$p(d^0, i^1 | g^3) + p(d^1, i^1 | g^3) = 0.08~~~~$ (given)
which happens to be 'incomplete', i.e. you can only collapse on an answer for all four if you arbitrarily assign a value to one of them and derive the rest ... which means we're still missing information. (Or, you can set one of those to 'x' and express the rest as a function of 'x').
EDIT(2):
Apologies, I was thrown off by the format of the table, which is a bit unusual; it is a table of conditional probabilities, as opposed to joint probabilities. For instance, cell $\{i^0d^0,g^3\}$ denotes the probability $p(g^3 | i^0d^0)$. Also, you know it's this probability rather than, say, $p(i^0d^0 | g^3)$ because the rows sum up to 1, i.e. given the row, the sum of the columns adds up to 1, and is therefore a complete probability distribution with respect to $g$ (given the row).
Given this information, we can now use the above system of equations, and the information from the table, namely:
\begin{array}{lll}
p(g^3 | i^0d^0) & = & 0.3 \\
p(g^3 | i^0d^1) & = & 0.7 \\
p(g^3 | i^1d^0) & = & 0.02 \\
p(g^3 | i^1d^1) & = & 0.2
\end{array}
and plug them into applications of the Bayes theorem.
Which still means these are all things to plug into a problem, as soon as a valid question is asked :)