How can I calculate the SD of a Gaussian obtained from sampling a binomial distribution

Question

I need to simulate some antibody labeling scenarios. To keep it non-field specific, I'll talk in terms of fruit instead of nanobodies though.

Let's say I have an infinite supply of fruit. 70% of this supply are apples. If I now sample this population an infinite amount of times, with a sample size of 24, I should get 24*0.7 apples on average in my samples. The distribution of fruit in these samples can (I think) be represented with a Gaussian distribution. My question is, is it possible to calculate the standard deviation of this distribution from only these two parameters? I think it should be, but have no idea how to do it.

Cheers!

Answer 1

Your question is not totally clear, but from what I understand you are drawing samples of size $n=24$ from the population where there is $70\%$ apples, so probability of drawing apple is $p=0.7$. The results can be described using binomial distribution. You are drawing $5000$ samples, each from binomial distribution, so their distribution is obviously binomial. We can approximate the results using normal distribution, but normal distribution is obviously not the correct distribution in here, as this is a discrete data, while normal distribution is a distribution for continuous values.

We do not need normal approximation in here at all, but if you want to know the variance, then we know that variance of binomial distribution is $np(1-p)$. Standard deviation is a square root of it.

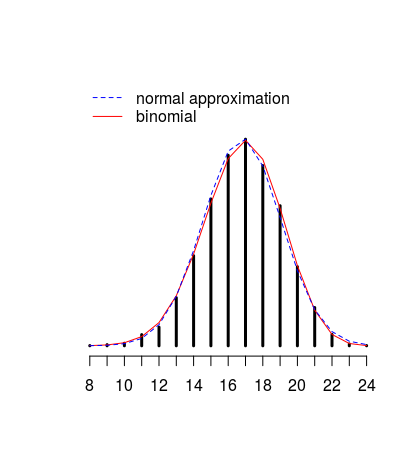
You can easily verify this by simulation, where we sample $5000$ values from the binomial distribution with parameters $n=24$ and $p=0.7$. The true binomial distribution function is drawn in red and the normal approximation in blue. As you can see, in this case normal approximation is very close to binomial and the variance calculated from the variance formula for binomial distribution is correct.

set.seed(123)

x <- rbinom(5000, size = 24, prob = 0.7)
tab <- prop.table(table(x))

plot(tab) # the code was simplified
lines(8:24, dbinom(8:24, size = 24, prob = 0.7), col = "red")
lines(8:24, dnorm(8:24, mean = 24*0.7, sd = sqrt(24*0.7*(1-0.7))),
      col = "blue", lty = 2)

Answer 2

Like Tim said, its distribution is Binomial and its variance = np(1-p). From your question this should be enough. You could approximate the Binomial distribution with the Normal distribution by the central limit theorem but would probably need more than 24 samples in each draw. The peak of the distribution will be at 0.7*24 = 16.8, not 70%. Don't confuse it with the Bernoulli distribution.