Interpreting quantile regression estimates

Question

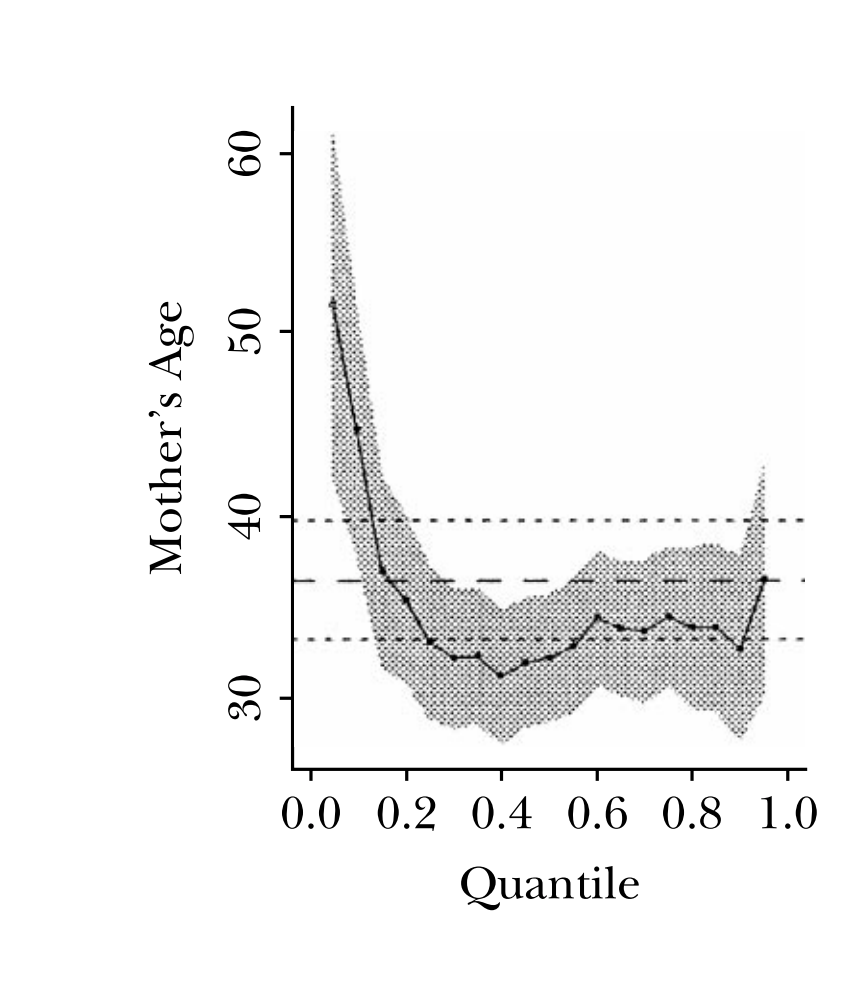
I am reading the paper of Roger Koenker about Quantile Regression. Specifically in Figure 4, I can see that at the lower quantiles, the effect of Mother's Age is strongest than at other higher quantiles, such as at the .1 quantile, 1 years old gained by mothers relates to about 45 grams gained by the baby. But how can the authors specifically interpret from the plot that

At the lower quantiles, the mother’s age tends to be more concave, increasing birthweight from age 18 to about age 30, but tending to decrease birthweight when the mother’s age is beyond 30

Answer 1

You first have to realize that the values on the y-axis are not age, but parameter estimates for mother's age. You next have to realize that (as the text shows) mother's age is a quadratic, so you have to look not just at this chart, but the one next to it in the paper.

At the 1st quantile, the parameter estimate for mother's age is about 50 and for age squared it is about -0.8, so, we have $50*Age - 0.8*Age^2$. Then you have to realize that age has been centered, so it ranges from about -20 to + 20. At the highest quantiles, it is more like $35*Age - 0.4*Age^2$.