1
$\begingroup$

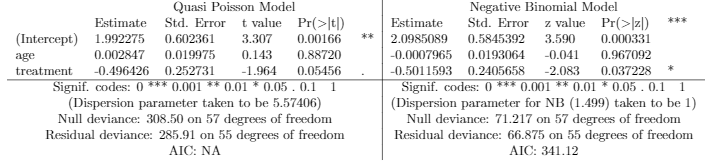
My data was over-dispersed (dispersion coefficient over 5), so I have fitted both the quasi-poisson model and the negative binomial model. I notice that the regression coefficients are almost the same, however the residual deviance varies a lot:

summary table from R

How can the difference in the residual deviance of both models be explained?

How can the difference in the residual deviance of both models be explained?

I want to check if the interaction effect between the two regressors is significant. Would any of the two model be better for including interaction? Which test statistic should be used in this case?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

The whole point of the quasi-distributions is to adjust for overdispersion via changing the weighs, which changes the deviance. Thus, you cannot compare quasi with other distributions via deviance or AIC, which is the reason why the AIC in the quasi models is suppressed.

Testing for interactions: just include an interaction in the model.

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.