Bravo @stans , auto.arima in a brute force list-based procedure that tries a fixed set of models and selects the calculated AIC based upon estimated parameters. The AIC should be calculated from residuals using models that control for intervention administration, otherwise the intervention effects are taken to be Gaussian noise, underestimating the actual model's autoregressive effect and thus miscalculates the model parameters which leads directly to an incorrect error sum of squares and ultimately an incorrect AIC. Most SE responders do not point out this assumption when they promote the free auto.arima tool which I think is a serious error of omission.
Modern/Correct/Advanced ARIMA time series analysis is conducted by identifying a starting model and then iterating to refine the initially suggested model as detailed in If I am convinced that a series is mostly trend+season, what is it I should check about the residuals? will be of help here.
If you wish please post your data I will illustrate this for you and the possible failings/omissions of auto.arima.
As an example of a very bad model identification using auto.arima see https://www.omicsonline.org/open-access/an-implementation-of-the-mycielski-algorithm-as-a-predictor-in-r-2090-4541-1000195.php?aid=65324 .
EDITED AFTER RECEIPT OF DATA:
I took your 200 observations and introduced them to AUTOBOX. Here is the original data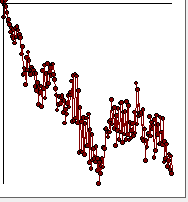 
. AUTOBOX automatically detected a change in model parameters at period 62 thus suggesting 2 regimes 1-61 and 62-200 which is (NOW !) visually obvious from the graph. This was based on the CHOW test for constancy of parameters.
The model is here 
with stats here 
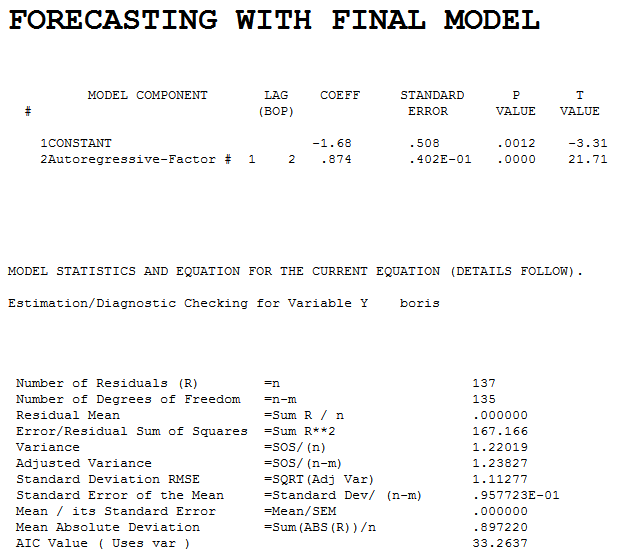. Note that there is 1 auto-regressive coefficient at lag 2 .
The Actual/Fit and Forecast is here 
with residual plot here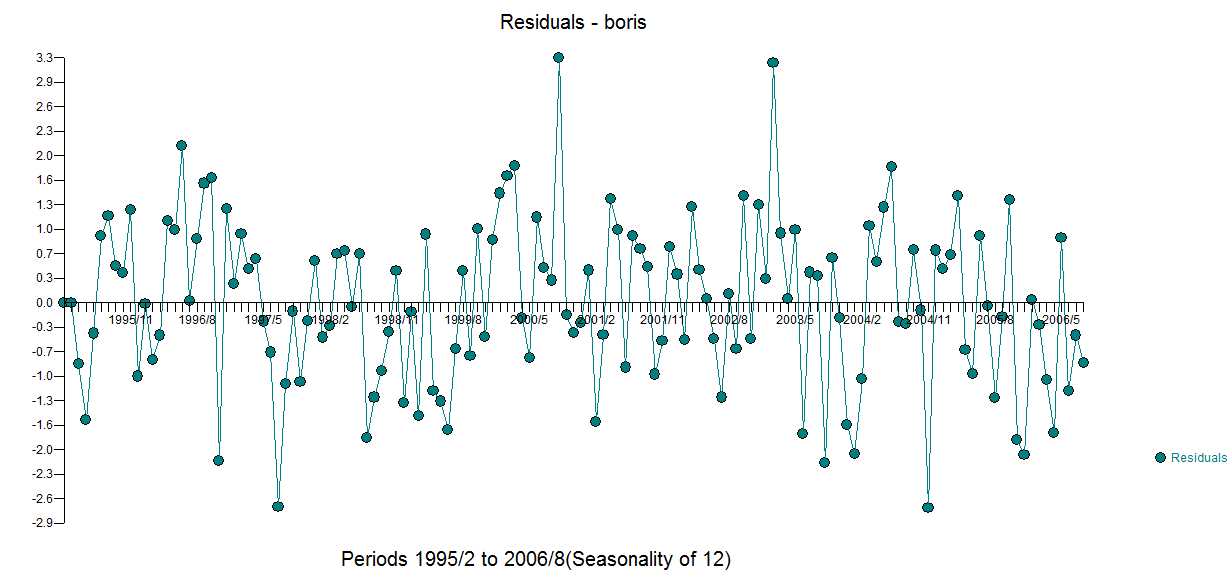 
and acf here 
suggesting model sufficiency.
The Fit and Forecast graph nicely presents the impact of the equation .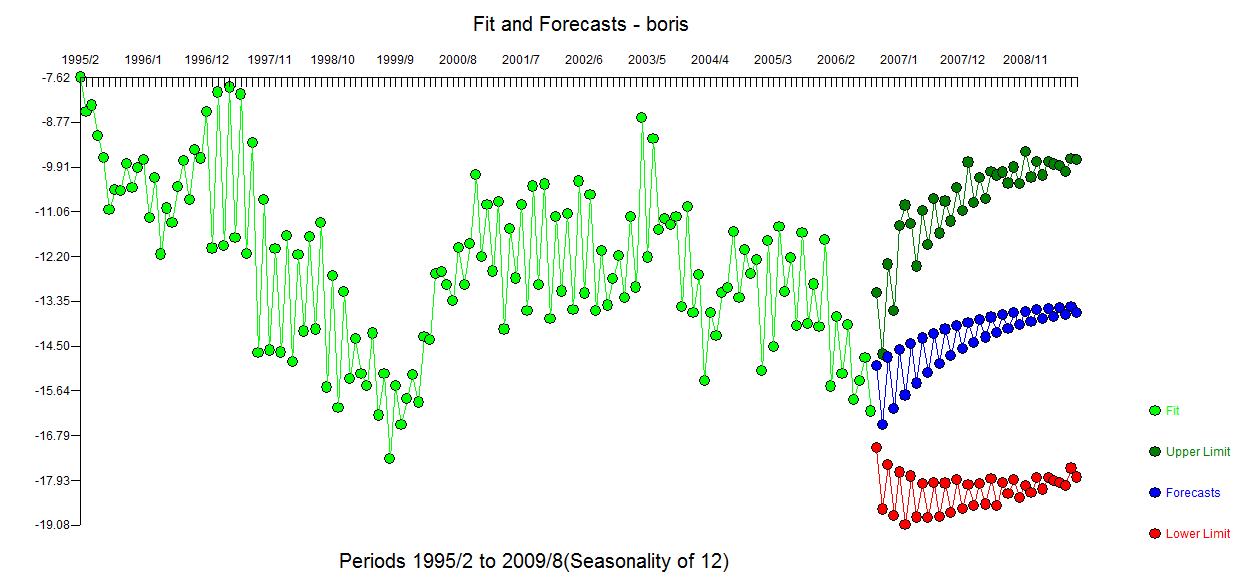
In summary the reason why your simple attempts to form a model with significant structure failed is that either the parameters changed at period 61 OR the error variance changed at period 62 creating OBFUSCATION in the acf yielding twice as much OBFUSCATION in attempting to torture/use the sample acf with a restricted set of models/solutions .
Is this simulated data ?
