Exact Poisson: First adjust the rate to 240 to match 12 hrs. Then let $X$ be the number
of calls in 12 hrs, where $X \sim \mathsf{Pois}(240)$ and you seek
$P(X = 250) = 0.0205.$ (Computation in R, but computation using the Poisson
PDF, or PMF, isn't difficult on a calculator.)
dpois(250, 240)
[1] 0.02053754
Normal approximation: You have $\mu = E(X) = 240$ and $\sigma^2 = Var(X) = 240.$ So
$\sigma = \sqrt{240} = 15.4919.$ So $$P(X = 240) \approx P(249.5 < Y < 250.5),$$ where $Y \sim \mathsf{Norm}(\mu = 240, \sigma=15.4919).$ Below is the computation of this normal
approximation using R. I suppose you are expected to get the answer by
standardizing and using normal tables. See how close you can get. [It may not be exact because a little rounding may be necessary to use printed normal tables.]
diff(pnorm(c(249.5, 250.5), 240, 15.4919))
[1] 0.02090658
Binomial approximation. Don't give up on using a binomial distribution. When $n$ is very large and $p$ is small, $\mathsf{Binom}(n, p)$ is well
approximated by $\mathsf{Pois}(\lambda = np).$ Let's try $n = 2400$ and $p = 1/10.$
dbinom(250, 2400, 1/10)
[1] 0.02120139
Or, maybe better, $n = 24,000$ and $p = 1/100.$
dbinom(250, 24000, 1/100)
[1] 0.02060194
Binomial probabilities can be a little messy to compute on a calculator because the factorials
in the binomial coefficient are so large.
The plot below shows the Poisson distribution (black bars, values between 230 and 260), the approximating normal density curve (blue), and the second binomial approximation (purple circles).
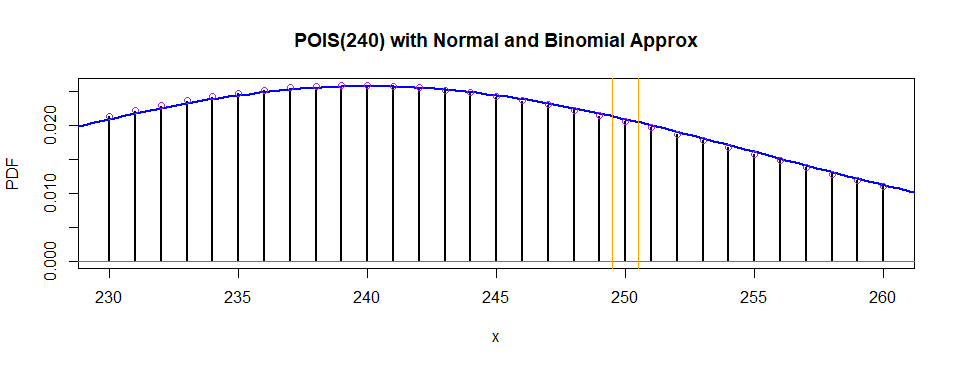
Note: In any case, it is useful to know relationships among binomial, Poisson, and normal distributions.

