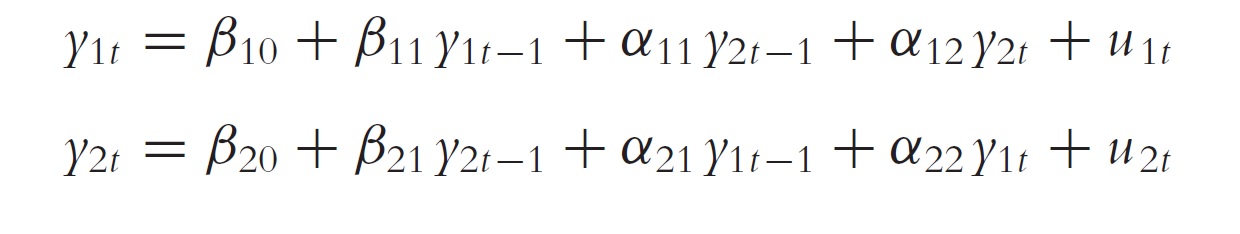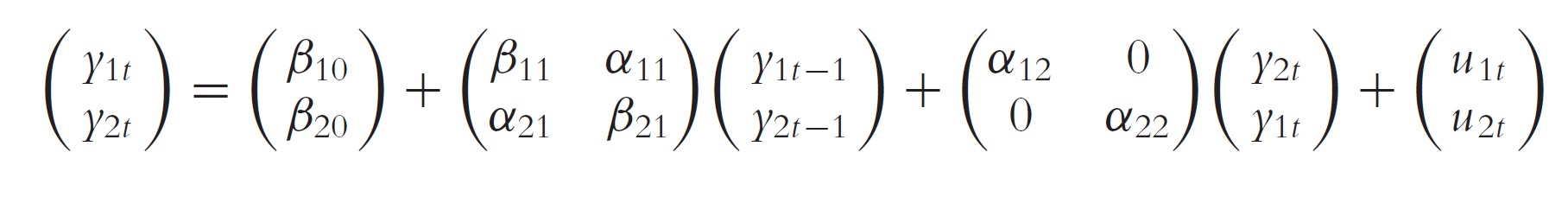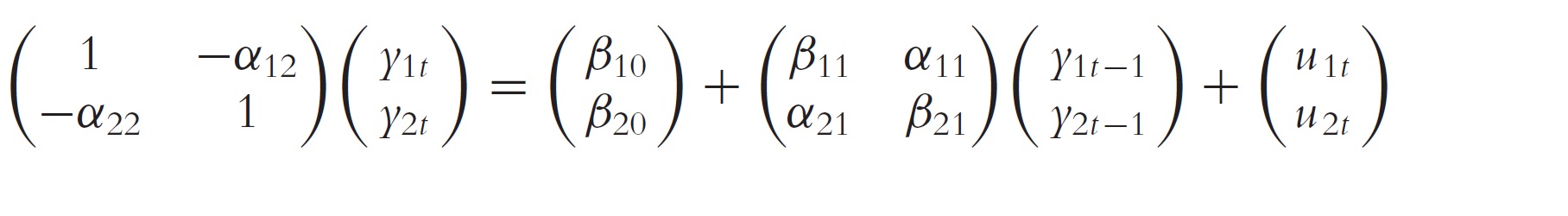Given a primitive form of a bivariate VAR(1) below,
and correspondingly in a matrix form below.
What were the steps involved in manipulating the matrices such that it resulted in this form below as the idea is to obtain a normal form VAR representation following these manipulations.
Just represent the vector as
$\left(\matrix{\gamma_{1,t}\\ \gamma_{2,t}\\}\right) = \left(\matrix{1&0\\0&1\\}\right) \left(\matrix{\gamma_{1,t}\\ \gamma_{2,t}\\}\right) $
then you just have to subtract the matrix with $\alpha_{i,j}$ effects from both sides and you get the matrix from the 2nd picture. Look that I manipulated the matrix and vector, because for some reason, the terms in the picture's vector are displaced.
$\left(\matrix{1&-\alpha_{1,1}\\-\alpha_{2,2}&1\\}\right)\left(\matrix{\gamma_{1,t}\\ \gamma_{2,t}\\}\right) = \left(\matrix{1&0\\0&1\\}\right) \left(\matrix{\gamma_{1,t}\\ \gamma_{2,t}\\}\right) - \left(\matrix{0&\alpha_{1,2}\\\alpha_{2,2}&0\\}\right)\left(\matrix{\gamma_{1,t}\\ \gamma_{2,t}\\}\right)$
