6
$\begingroup$

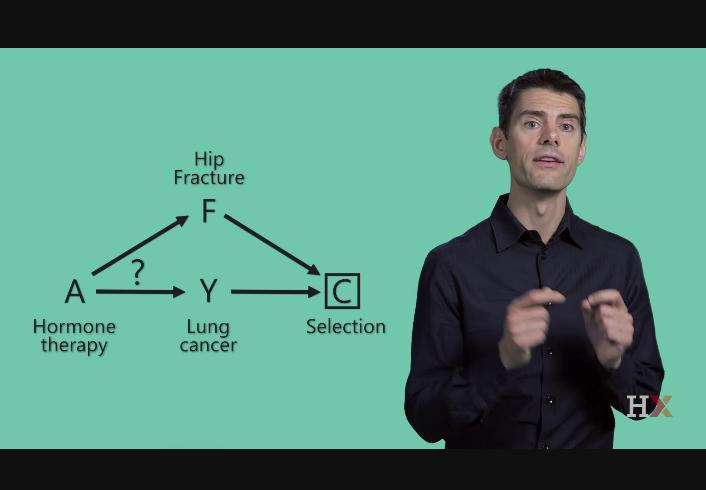
In Lesson 3, Chapter 3 of Miguel Hernán's edX course on causal diagrams, he presents this DAG:

enter image description here

It represents a study on the effect of hormone therapy on lung cancer (whether hormone therapy causes lung cancer). Among women with lung cancer in Boston, a random sample of 1,000 has been selected for the study. Among women without lung cancer in Boston, a random sample of 1,000 has also been selected for the study. This accounts for the arrow between lung cancer and selection (i.e. outcome-based selection).

Then he adds that only women with hip fractures were surveyed ("they can't run away from you"), which accounts for the arrow between hip fracture and selection.

Finally, he says that hormone therapy actually reduces the risk of hip fracture, so the final arrow between hormone therapy and hip fracture is added, creating an open backdoor path.

My question is, if only women with hip fractures were surveyed, doesn't that mean we are conditioning on hip fractures? If so, there should be a square around hip fracture, the backdoor path is actually blocked, and there is no selection bias.

Improve this question
$\endgroup$
3
  • $\begingroup$ What is the objectives of this study? Effect means fracture? $\endgroup$
    – user158565
    Commented Nov 6, 2018 at 16:42
  • $\begingroup$ The effect in question is the effect of hormone therapy on lung cancer (whether hormone therapy causes lung cancer). $\endgroup$
    – suckrates
    Commented Nov 6, 2018 at 16:50
  • $\begingroup$ Maybe I need to see the video when I have time. $\endgroup$
    – user158565
    Commented Nov 6, 2018 at 16:56

2 Answers 2

Reset to default
5
$\begingroup$

If it's true that only women with a hip fracture were selected, then there is no association between hip fracture and anything in the selected population. This would amount to saying something like "among women with hip fractures, there is an association between having a hip fracture and having lung cancer." Clearly, this doesn't make sense. An association requires variation in two variables. You can't talk about an association between a variable and a conditioned-upon variable.

If what he meant was "women with hip fractures were more likely to be selected," then one is not conditioning on hip fracture but rather on selection, which is caused by fracture, as the DAG displays. He may have misspoken in the video, because this is clearly what he intended to mean.

Improve this answer
$\endgroup$
1
  • 4
    $\begingroup$ You are right. I listened to the video again and he says "we are going to select MOST of our controls" among women that have had hip fractures. So women that have had hip fractures are only more likely to be selected, not deterministically selected like I understood. $\endgroup$
    – suckrates
    Commented Nov 6, 2018 at 17:08
4
$\begingroup$

My question is, if only women with hip fractures were surveyed, doesn't that mean we are conditioning on hip fractures? If so, there should be a square around hip fracture, the backdoor path is actually blocked, and there is no selection bias.

Notice that even if the DAG were only $A \rightarrow Y \rightarrow C$ the post-interventional distribution $P(Y|do(A))$ is not non-parametrically identified, since $P(Y|do(A)) = P(Y|A) \neq P(Y|A, C)$ and you only observe $P(Y|A, C =1)$. Thus, if your target estimate is $P(Y|do(A))$, there is selection bias even without the path $A \rightarrow F \rightarrow C$.

For selection bias, you might want to check Bareinboim and Pearl and more recently Correa, Tian and Bareinboim.

Improve this answer
$\endgroup$
4
  • $\begingroup$ I think I see what you're saying. Then why does he make so much emphasis on selection being a common effect of treatment and outcome for there to be selection bias? He could have just stopped at $A \rightarrow Y \rightarrow C$ $\endgroup$
    – suckrates
    Commented Nov 6, 2018 at 19:17
  • $\begingroup$ Could it be because he is only teaching setups where there can be bias under the null? $\endgroup$
    – suckrates
    Commented Nov 6, 2018 at 19:27
  • 1
    $\begingroup$ @AlvaroFuentes I don't know, I will ask Hernan. But you can be assured that there's selection bias regardless of $F$ in this case here. I will include some references on my answer as well. $\endgroup$
    – Carlos Cinelli
    Commented Nov 6, 2018 at 19:31
  • 2
    $\begingroup$ @AlvaroFuentes If you read Hernán & Robins' Causal Inference, Chapter 8: Selection Bias, you will see that their definition of the causal structure of all selection bias is that you are conditioning your association between $A$ and $Y$ on a common descendant of both $A$ and $Y,$ or on a variable associated with such a descendant. $\endgroup$
    – Alexis
    Commented Mar 15, 2019 at 17:23

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.