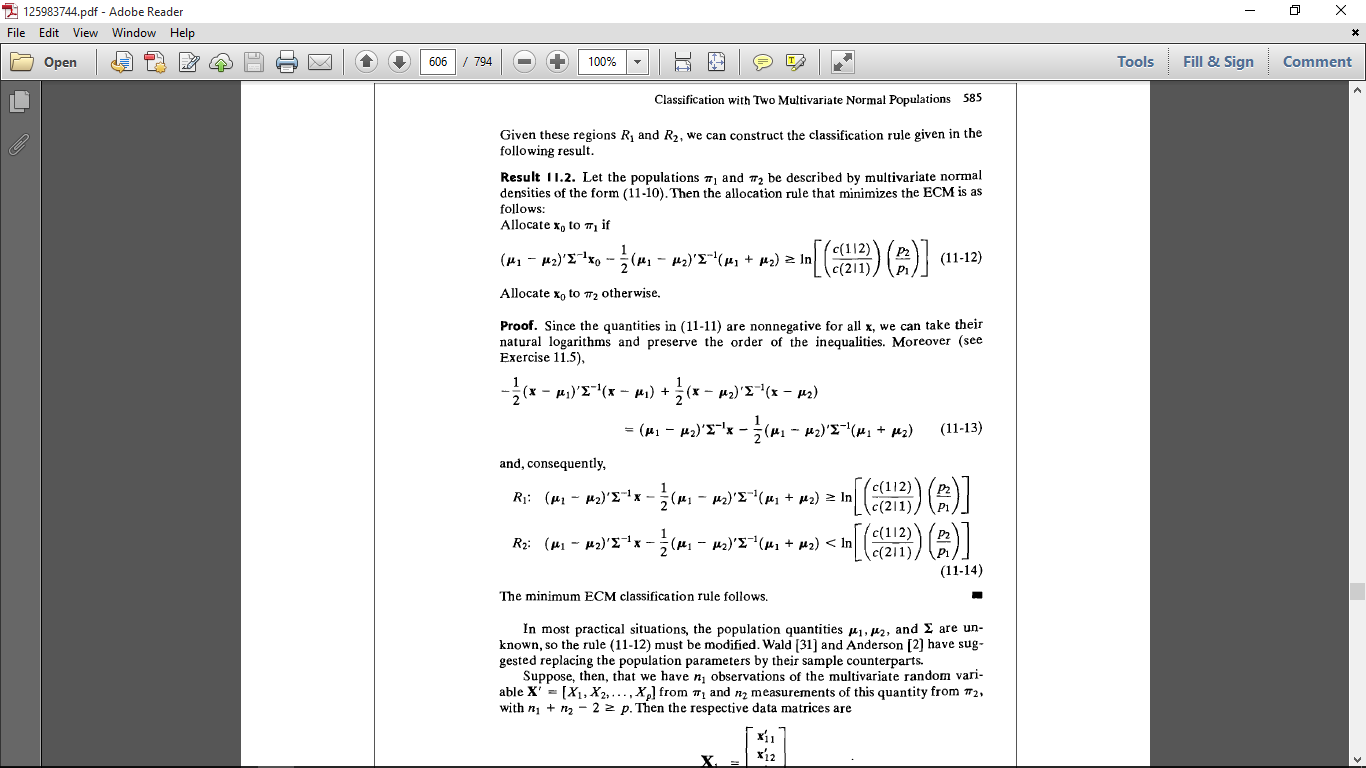
this is related to quadratic discriminant analysis. I want to prove the 11-13 using the result 11.2 as shown in the image.Can anyone suggest the steps to complete the proof?
$\begingroup$
$\endgroup$
1
-
$\begingroup$ The question asks you carry out "exercise 11.5." Why not do that? $\endgroup$– whuber ♦Commented Apr 23, 2019 at 15:00
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
1
Note that $B(x,y):=\frac12x'\Sigma^{-1}y$ is a symmetric bilinear form, therefore: $$-\frac{1}{2}(x-\mu_1)'\Sigma^{-1}(x-\mu_1)+\frac{1}{2}(x-\mu_2)'\Sigma^{-1}(x-\mu_2)$$ $$=-B(x-\mu_1,x-\mu_1)+B(x-\mu_2,x-\mu_2)$$ $$=-B(x,x)+B(x,\mu_1)+B(\mu_1,x)-B(\mu_1, \mu_1)+B(x,x)-B(x,\mu_2)-B(\mu_2,x)+B(\mu_2, \mu_2)$$ $$=2B(\mu_1,x)-2B(\mu_2,x)-B(\mu_1,\mu_1)+B(\mu_2,\mu_2)$$ $$=2B(\mu_1-\mu_2,x)+B(\mu_2-\mu_1,\mu_2+\mu_1)$$ $$=2B(\mu_1-\mu_2,x)-B(\mu_1-\mu_2,\mu_1+\mu_2)$$ $$=(\mu_1-\mu_2)'\Sigma^{-1}x-\frac{1}{2}(\mu_1-\mu_2)'\Sigma^{-1}(\mu_1+\mu_2)$$
-
$\begingroup$ Thank you very much for your help. I really appreciate it $\endgroup$ Commented May 30, 2019 at 14:24