I have a normal random variable $X$ with mean $\mu$ and variance $\sigma^2$. Any advice on how to compute the conditional expectation $E[\frac{1}{X}|X \leq T]$ where $T$ is a positive constant?
$\begingroup$
$\endgroup$
5
-
1$\begingroup$ this link looks relevant. stats.stackexchange.com/questions/70045/… $\endgroup$– mloftonCommented Aug 14, 2019 at 4:56
-
2$\begingroup$ As with the link above, the expectation will not converge, given the 1/X and the domain of support including 0. $\endgroup$– wolfiesCommented Aug 14, 2019 at 6:52
-
$\begingroup$ Because this conditional variable has positive continuous density in a neighborhood of zero, stats.stackexchange.com/questions/299722 demonstrates the expectation does not exist. $\endgroup$– whuber ♦Commented Aug 14, 2019 at 13:02
-
$\begingroup$ @whuber Yes, I saw some of those links before, but if the mean is sufficiently large, would that make it okay? Would simulation be the best way to approximate this? $\endgroup$– dotpixelCommented Aug 14, 2019 at 16:49
-
$\begingroup$ No, it doesn't help. My analysis (in one of those answers) shows the result has nothing to do with the mean. It's all about the fact that there's sufficient probability for $X$ to be close enough to zero that no expectation can exist. This will be true of any Normal distribution (although, to be sure, as a practical matter that probability may be negligible: but that's a different question). $\endgroup$– whuber ♦Commented Aug 14, 2019 at 18:57
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
2
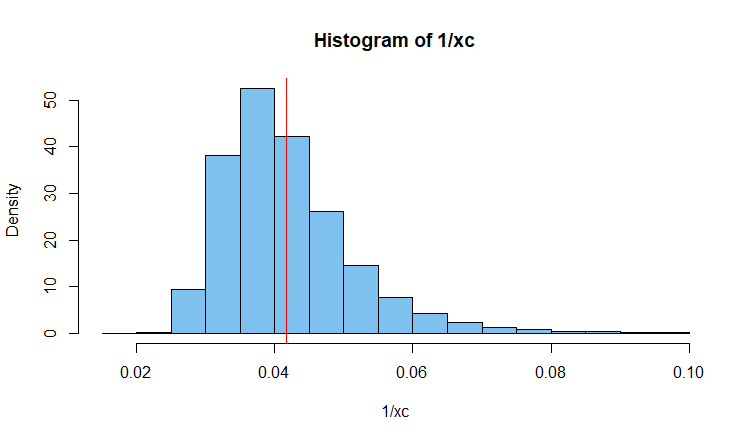
Comment: Simulation for $T = 10,$ which avoids taking reciprocals of values anywhere near $0.$ Then $E(\frac 1 X\, |\, X > 10) \approx 0.042.$ (As @Wolfies comments, this is a different problem.)
set.seed(2019)
x = rnorm(10^6, 25, 5)
xc = x[x > 10]
length(xc)
[1] 998638
a = mean(1/xc); a
[1] 0.04174508
hist(1/xc, prob=T, col="skyblue2")
abline(v=a, col="red")
Tangentially related application: Here
-
2$\begingroup$ $X>T$ (for T positive) is not the same as $X < T$, when considering $\frac1X$ $\endgroup$– wolfiesCommented Aug 14, 2019 at 7:07
-
$\begingroup$ Right: Should have noted explicitly that I was changing the rules to get an expectation that exists. Editing. $\endgroup$– BruceETCommented Aug 14, 2019 at 7:16