1
$\begingroup$

I am currently doing local sensitivity analysis on a model that has 40 input parameters. I varied the base case value by 10% within the range of [-1, 1]. While doing so the average change (here, I averaged the values for all observations and got the single output) with the base case value is 0 for some parameters.

How do I interpret 0 in this case? Does this mean that the parameters I am using have no influence in my output?

I could not find any literature that could verify my interpretation. Hence, any help/suggestion would be highly appreciated.

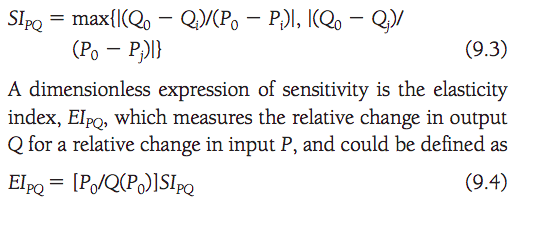
The equations I used to calculate sensitivity index (SI) and elasticity (EI) is given below: Q being the output and P being the input to the model. SI AND EI

Thank you!

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

First answer would be: yes, probably they have no influence. But to be sure you should tell us how you compute your sensitivity index... You should also check Saltelli's papers in the scientific literature. With 40 parameters, you should probably use global rather than local SA, because your parameters probably interact (that is the sensitivity to a parameter may depend on the value of other parameters). You will miss this kind of effect and over- or under-estimate sensitivity with just a local analysis.

Improve this answer
$\endgroup$
1
  • $\begingroup$ That is true. Local SA completely disregards the interaction effects. I am considering performing variance-based SA as well but I wanted to first determine which indicators would have a huge influence on the index locally and compare the result later with the variance-based SA [having difficulty to understand this as well]. I performed local SA by changing the raw values by equal interval (10%, 20% ,...and so on) and calculated the corresponding output and used these values to calculate the sensitivity index and elasticity. I have added the equation I used in my question above. $\endgroup$
    – tropicalbath
    Commented Sep 6, 2019 at 13:06

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.