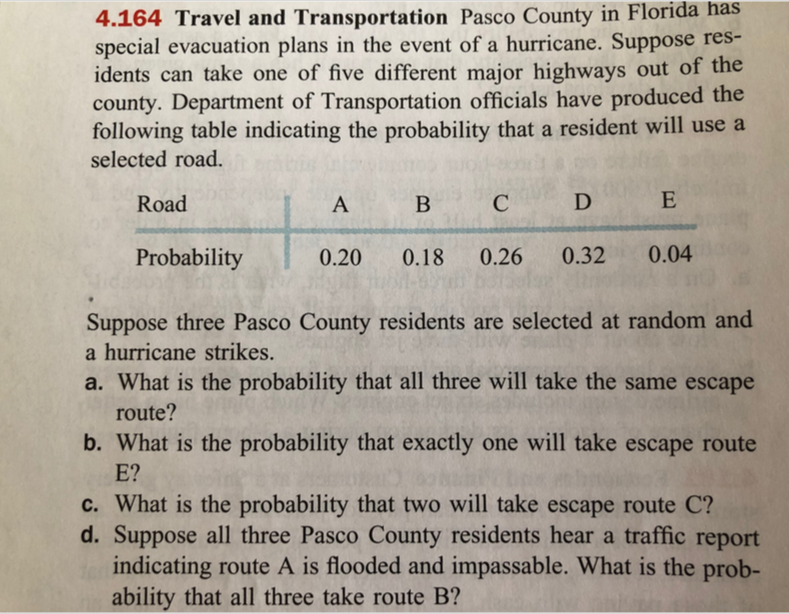
This is a question from my textbook, there is no answers at the back and I am fairly new to statistics, my answers for questions a-d are below, can anyone check if I answered them correctly?
a) All Pick $A: (0.20)(0.20)(0.20)= 0.008$
All Pick $B: (0.18)(0.18)(0.18)= 0.005832$
All Pick $C: (0.26)(0.26)(0.26)= 0.017576$
All Pick $ D: (0.32)(0.32)(0.32)= 0.032768$
All Pick $E: (0.04)(0.04)(0.04)=0.000064$
Sum$= 0.008+0.005832+0.017576+0.032768+0.000064=0.06424$
Probability$= (0.06424) \times 100\% = 6.42\%$
b) $(0.04)(0.96)(0.96)= 0.036864 $
Probability $= (0.036864) \times 100\% = 3.69\%$
c) $(0.26)(0.26)(0.74) = 0.050024$
Probability $= (0.050024) \times 100\%= 5.00\%$
d) Sum of probability $= 1$
New sum of probability$= 1-0.20 = 0.80$
New probability of $B = \frac{0.18}{0.80}= 0.225$
All $3$ take $B$: $(0.225)^3 \times 100\% = 1.14\%$