why can two random variables be added only when they have the same domain?

Question

I am watching lecture 7 in harvard stats 110 and the professor is teaching distribution of addition of two random variables and in a breadth says that random variables can be added only if their domains are same. Which i don't understand completely. Why can i not see by just addition, how $X+Y$ is distributed if $X \sim Bin(n,p)$ and $Y \sim N(\mu,\sigma)$? $X$ and $Y$ can be random variables on completely different sample spaces like $X$ being the number of heads in N coin tosses and $Y$ being excess return on some index

Answer 1

For me this quora post seems to answer it

A random variable is a way of labeling the outcomes of an experiment with a real number. You can think of it as attaching every outcome with a label, the label being a real number. For instance, if an experiment has two outcomes ω1,ω2, so that the sample space is Ω={ω1,ω2}, we can ‘attach’ a label to each of the two outcomes. For example, we could label the outcomes with 1,π. This, in an informal sense, is a random variable. It is completely possible to assign one another set of labels, say −7/8,2–√, for ω1,ω2. This will correspond to a second random variable. The sum of above random variables is another pair of labels, with the label being the sum of original labels. Thus a new pair of labels is 1−7/8,π+2–√, which, again in an informal sense, is the sum of two random variables.

To put this in a formal way, a random variable X:Ω→R is a function from the sample space to the set of real numbers. If X1:Ω→R,X2:Ω→R are two random variables, then their sum X1+X2:Ω→R is a function defined by X1+X2(ω):=X1(ω)+X2(ω). The sum of many random variables is defined in a similar manner. Are we done? Well, not yet! In many cases, the random variables are defined on different sample spaces and we want to add them. How do we do that? Since random variables are functions, they must have same domain to be able to add them. Thus we construct a product space Ω:=Ω1×Ω2, and hence every ω∈Ω can be written as ω=(ω1,ω2) for ω1∈Ω1,ω2∈Ω2. We also define X1(ω):=X1(ω1),X2(ω):=X2(ω2). Now we can add the two random variables just like functions as they have the same domain. Similarly, for n random variables we construct an n−fold product ∏1≤i≤nXi and define their sum. This would be become more clear to you once you construct your own random variables and start playing around with them.

Also, i found the link provided in whuber's comment on question very useful

Answer 2

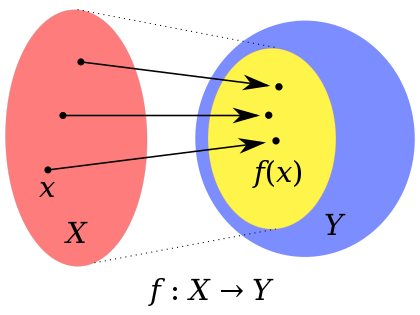
You misunderstood the meaning of domain, see Wikipedia which have the following figure:

In that figure the domain is X, and Y is the codomain (or range or target space.) For the sum to be defined it is clear that the codomains (of the $X$ and $Y$ in your example) must be compatible, in being (subsets of) some numerical space where addition is defined, no problem in your example. But that is about the codomains.

The domain for a random variable (which really is a function, see What is meant by a "random variable"?) is a probability space. The probability space in probability theory is, intuitively, the source of randomness. What should the meaning of $X+Y$ as a random variable be if the sources of randomness in $X$ and $Y$ where distinct? Or simply look at the formal definition of sum of functions which do not make sense if the domains are distinct.

Answer 3

Simply put, they need to have dimensional homogeneity (https://en.wikipedia.org/wiki/Dimensional_analysis#Dimensional_homogeneity) but the domain doesn't need to be exactly the same.

Imagine one random variable refers to the position and another to time. Obviously, you can't just add up those in any meaningful manner (even if both share the same domain, namely the real numbers). Another example is to consider the result of tossing a coin (head or tails) and add it to the result of rolling a dice (1, 2, 3, 4, 5 or 6), again meaningless. On the other hand, you can legitimately add two random variables even if the domain differs, for example, if you have 2 uniformly distributed variables with non-overlapping domains (i.e, one in [0,1] and the other in [4,5]). In this case, their domains are different but they can be added as long as they have the same units. In summary, to add up 2 random variables the domain doesn't need to be the same, but the add operation must make sense for which it is required dimensional homogeneity.

Hope it helps.