0
$\begingroup$

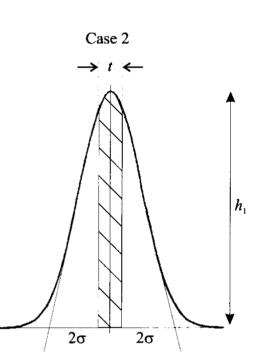
I am interested in a nice function to find the area of a section of a normalized Gaussian distribution. Let's say I have a normalized Gaussian distribution of standard deviation $\sigma$, mean $\mu$, and I am interested in the area centered at $\mu$ between +/- $t$ ( see below) enter image description here

Is there a simple way to express this for any given $t$ using the erf? The ref from which I found the above figure uses

$$a_1 = 2 \mathrm{erf}\left(\frac{t}{\sigma} \right) $$

But I don't think this expression is true for a normalized guassian. Moreover, can the $\textit{total}$ area be expressed by an error function?

Thanks, very new to statistics.

Improve this question
$\endgroup$
2
  • $\begingroup$ Which quantities in the diagram are 'known'? $\endgroup$
    – Glen_b
    Commented Nov 17, 2019 at 2:41
  • $\begingroup$ all of them- t, sigma, and h are all known. $\endgroup$
    – Jimbo
    Commented Nov 18, 2019 at 5:38

1 Answer 1

Reset to default
0
$\begingroup$

This question has already been answered here. Note that you can compute the integral over a finite interval by means of the CDF $\Phi$: $$\int_a^b \varphi(x)\,dx = \Phi(b) - \Phi(a)$$

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.