4
$\begingroup$

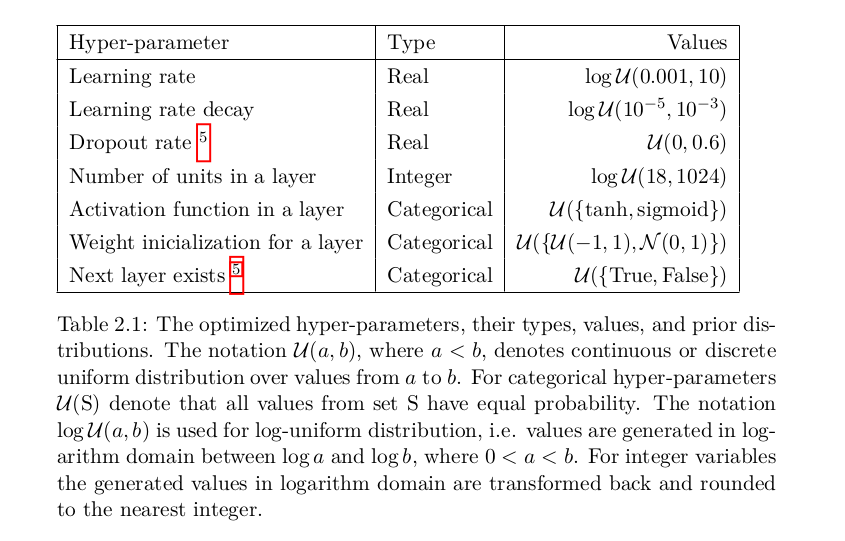
I am wondering how did they get the $19200$ possible configurations? Like, $5^6 = 15625$, where $6$ is the number of hyper-parameters:

enter image description here

ps: just to check I'm doing the righ thing. Is this okay? The formula is $[a,b]: b -a + 1$. So in the second screenshot, the total number would be $31 \times 71 \times 71\ \times\ 9 \times 9 = 12.657.951$ possible configurations?

enter image description here

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
5
$\begingroup$

Each hyper parameter listed has $5,5,6,4,4,8$ different values listed. If multiplied, total number of combinations make $5\times5\times6\times4\times4\times8=19200$.

Improve this answer
$\endgroup$
13
  • $\begingroup$ Thank you very much! They are including the values from the extremes i.e., [2,7], 2 and 7 are included. $\endgroup$
    – Stephen
    Nov 23, 2019 at 19:35
  • $\begingroup$ Yes, typically brackets $[,]$ means the boundaries are included. $\endgroup$
    – gunes
    Nov 23, 2019 at 19:36
  • 1
    $\begingroup$ Yes, that's the correct formula. $\endgroup$
    – gunes
    Nov 23, 2019 at 19:55
  • 1
    $\begingroup$ Check the updated OP. I added another screenshot $\endgroup$
    – Stephen
    Nov 23, 2019 at 20:20
  • 1
    $\begingroup$ It's always good to be able to reduce the number of possibilities, or ranges if you have continuous variables. Bayesian HPO can do better in short time if you do. $\endgroup$
    – gunes
    Nov 24, 2019 at 20:12

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge that you have read and understand our privacy policy and code of conduct.

Not the answer you're looking for? Browse other questions tagged or ask your own question.