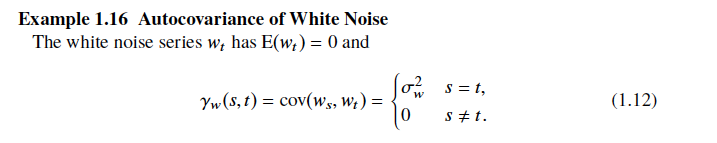1
$\begingroup$

First of all I am a newbie in stats, trying to study statistics, and somehow I find 'time series' way more difficult than other topics.

I am confused with the “autocovariance”. In the book "Time series analysis and its applications", it says:

enter image description here

Before my question I must admit that I did not know what values of $w_s$ and $w_t$ shall be chosen, and what I understood from my previous question is that you have to specify a lag: logic of autocovariance in time series

ie: lag 1 is to compare each value with the one right before.

But now I have 2 questions:

  • Why the author never defines the lag he is using? is he calculating the autocovariance for lag 1,2? or what?
  • I do not see how the covariance would be 0... If I think of the case for lag 1, in my head I picture potential values like: -0.5, 1, -2, 1, 1.

I see that the autocovariance will be negative (one value goes up, the next one goes down...)

Of course I do know my logic is faulty, the author shall not be wrong, but I want to fully understand it before proceeding to other topics... How come it is 0?

Improve this question
$\endgroup$
2
  • $\begingroup$ If $s=t$ the lag is 0, both $s$ and $t$ is time index so when given that $s=t$ then $cov(w_s,w_t) = cov(w_t,w_t) = var(w_t)$. If they are different $s\not=t$ the lag is different from 0 and hence you are looking at different timeperiods, but a white noise process is per definition one where there is no correlation across time that is where $cov(w_s,w_t) = 0 $ follows from $s \not = t$. $\endgroup$
    – Jesper for President
    Commented Nov 25, 2019 at 16:30
  • 1
    $\begingroup$ The lag is $s-t.$ By fiat the covariance is zero for nonzero lags. $\endgroup$
    – whuber ♦
    Commented Nov 25, 2019 at 16:45

1 Answer 1

Reset to default
1
$\begingroup$

In the example they refer to white noise time-series, so the "random" one, where there is no correlation by definition, since the values are independent of each other.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.