4
$\begingroup$

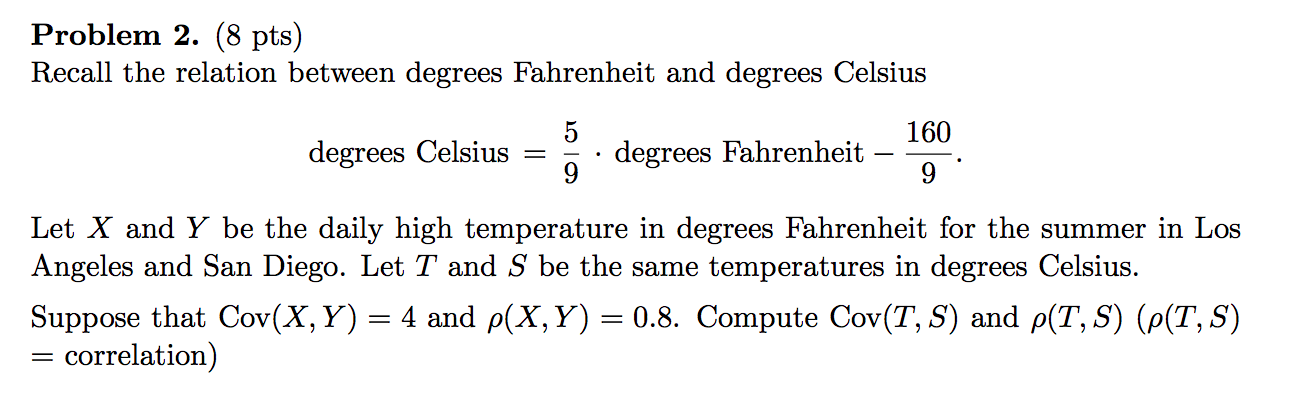
problem set

I know that correlation coefficient is scale invariant but my math says otherwise. By calculation, I got that $$\operatorname{std}(x) \operatorname{std}(y) = 5.$$ So by squaring both sides, $$\operatorname{Var}(x)\operatorname{Var}(y) = 25.$$ Then by using $\operatorname{Var}(aX) = a^2\operatorname{Var}(X)$, I got $$\frac{625}{6561} \cdot \operatorname{Var}(t)\operatorname{Var}(s) = 25.$$ Hence $\operatorname{Var}(t)\operatorname{Var}(s) = \frac{6561}{25}$. Then by square rooting both sides, $\operatorname{std}(t)\operatorname{std}(s) = \frac{81}5$. From the first part of the solution, $\operatorname{Cov}(t,s) = \frac{100}{81}$ so $\rho(t,s) = \frac{100}{729}$ which is $\neq \rho(x,y) = 0.8$. But they are supposed to be the same because correlation is scale invariant right? Where did I go wrong?

I found out that my math is in fact not wrong. I used $X = \frac{5}{9} T$ when it should have been $X = \frac{9}{5} T$ . After rectifying that, I got the answer using my method!

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ This question would be easier to read using math formatting. math.meta.stackexchange.com/questions/5020/… $\endgroup$
    – Sycorax ♦
    Commented Jan 13, 2020 at 1:54
  • $\begingroup$ You can answer these questions with minimal calculation and no algebra, using only the insights (from simple pictures) derived at the end of stats.stackexchange.com/a/18200/919. They show that $\rho$ will not change and the covariance will be multiplied by $(5/9)^2.$ $\endgroup$
    – whuber ♦
    Commented Jan 13, 2020 at 18:35

1 Answer 1

Reset to default
11
$\begingroup$

$\newcommand{\Cov}{\operatorname{Cov}}$The multiplication rule for covariances is $\Cov(aX,bY)=ab\Cov(X,Y)$. Note that the constant term ($-\frac{160}{9}$) doesn't affect the standard deviation or covariances of the temperatures, so we can ignore it. Since $T=\frac{5}{9}X$ and $S=\frac{5}{9}Y$, we know that $$\Cov(T,S)=\Cov\left(\frac{5}{9}X,\frac{5}{9}Y\right)=(\frac{5}{9})^2 \Cov(X,Y)=\left(\frac{5}{9}\right)^2 4$$

The multiplication rule for standard deviations comes from the rule for variances: $s_{kX}=ks_X$. So, $$\rho(T,S)=\frac{\Cov(T,S)}{s_T s_S}=\frac{\Cov(\frac{5}{9}X,\frac{5}{9}Y)}{s_{\frac{5}{9}X}s_{\frac{5}{9}Y}}=\frac{(\frac{5}{9})^2 \Cov(X,Y)}{(\frac{5}{9})^2 s_X s_Y}=\frac{\Cov(X,Y)}{s_X s_Y} = \rho(X,Y)=0.8$$

Improve this answer
$\endgroup$
2
  • 3
    $\begingroup$ You probably want $\text{ Cov}(aX+c,bY+d)=ab\text{ Cov}(X,Y)$ and $T=\frac59X-\frac{160}{9}$ etc before you ignore the constant, though this does not affect the second half of what you wrote $\endgroup$
    – Henry
    Commented Jan 13, 2020 at 11:21
  • $\begingroup$ Good point, I was just being lazy. $\endgroup$
    – Noah
    Commented Jan 13, 2020 at 15:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.